Jacques Brel

Gay Marshall

all were children like your own

Sons of

Sons of the thief, sons of the saint
Who is the child with no complaint
Sons of the great or sons unknown
All were children like your own
The same sweet smiles, the same sad tears
The cries at night, the nightmare fears
Sons of the great or sons unknown
All were children like your own...
So long ago: long, long, ago...
But sons of tycoons or sons of the farms
All of the children ran from your arms
Through fields of gold, through fields of ruin
All of the children vanished too soon
In tow'ring waves, in walls of flesh
Among dying birds trembling with death
Sons of tycoons or sons of the farms
All of the children ran from your arms...
So long ago: long, long, ago...
But sons of your sons or sons passing by
Children we lost in lullabies
Sons of true love or sons of regret
All of the sons you cannot forget
Some built the roads, some wrote the poems
Some went to war, some never came home
Sons of your sons or sons passing by
Children we lost in lullabies...
So long ago: long, long, ago
But, sons of the thief, sons of the saint
Who is the child with no complaint
Sons of the great or sons unknown
All were children like your own
The same sweet smiles, the same sad tears
The cries at night, the nightmare fears
Sons of the great or sons unknown
All were children like your own...
Like your own, like your own

Jacques Brel is Alive and Living in Paris

sung by Gay Marshall
Ed heard her sing at the French consulate. We saw her tonight in Jacques Brel.

Beautiful.

'Sons of' Today ...

excellent schools

I curse the person who invented disaggregated data.

Today we define excellent schools as ones that suck the least.

An improving school is one that can limit the drop off in performance in students as they get older the most.

A good teacher is one who can get their kids' parents to do the most teaching.

If the education system was a corporation, it would be Enron.

If the education reform was a soft drink, it would be New Coke.

question for the instructivist

Instructivist left this comment:

When I was teaching DI to 8th graders some of the hurdles were understanding that conversion factors like 1 ft/12 in mean one and that we are taking advantage of the identity element for multiplication. Another hurdle was to figure out which unit of the CF should be in the numerator and vice-versa. I thought I had developed crystal-clear strategies for a foolproof approach, even though the approach didn't sink in easily.

I always insisted on writing out each step and wrote the direction of the conversion on top of the problem with an arrow to minimize confusion, e.g. sec --> hours. Not all students were converts to my approach to conversion.

I don't quite get the arrow part.

I wish to heck I'd kept more notes on dimensional analysis.

Saxon teaches dimensional analysis throughout all his books, starting maybe in 7-6.

Several times I've thought I had it down cold, and then encountered a problem that stumped me.


notes:

• every single time we work on dimensional analysis I say to Christopher: "What does 1 ft/12" equal? Then I wait 'til he tells me it equals 1. Sometimes he doesn't tell me it equals 1, so I tell him. Then I say, "Why can we multiply the initial value by 1 ft/12"? That he always gets: we're multiplying by 1. Then I say, "Have we changed this initial amount? Is it a different amount after we've done all this multiplying by unit multipliers?" He gets that one, though he's slightly hesitant.... "...No..." Finally I say, "What has changed?" He may or may not say that the unit has changed, but that's only because he's not necessarily following my train of thought. As soon as I say it he gets it. I've become a huge fan of scripted instruction. I do this script every time we work on unit multipliers; when Christopher reaches the point where the script seems stupid and obvious to him I'll know he's got them conceptually as well as procedurally. (Or at least that he's got a far more solid conceptual understanding than he did when we started out.)
  

• Christopher has no trouble figuring out which unit has to go in the numerator and denominator, and neither did I including back when I first learned unit multipliers. I think there's something visual about it (and I believe visual memory is "stickier" though I have yet to review all that research).
  

• He sometimes gets confused about which number is which: for a particular problem he'll know he has to put yards in the numerator and feet in the denominator, but he'll write 3 yards/1 ft because 3-to-1 makes more sense or is more familiar (you probably know what I mean). So, although he has zero confusion about the canceling aspect of unit multipliers, the very fact that sometimes yards will be in the numerator and sometimes they'll be in the denominator can trip him up.

• I had a bit of trouble moving from "easy" unit multipliers (centimeters to yards) to rate unit multipliers (mph to meters per second), but Christopher has had no trouble at all. I'm sure that's because Christopher still writes the number 1 in the denominator of the rate: 60 miles/1 hour. I wish I'd thought of that. For quite awhile I kept thinking things like, "Wait! I have two units in the numerator! (60 mph - it's all one chunk) What do I do now!" This is one of those times where having a fresher brain is an advantage.

• I think the single hardest aspect of unit multipliers is knowing which number to put first. To this day I don't quite know whether it matters; I've gotten jumbled up in long problems before and had to unjumble myself by deciding there was one, and just one, number that could start the whole thing out. When Christopher reads a simple unit multiplier word problem I have him circle the value to be translated and underline the unit he's supposed to end up with. He's not particularly interested in doing that, but on the other hand the fact that we have done it seems to have made it fairly easy for him to figure out where to start.

• I have him do the cancellations as he goes along. I learned this the hard way. By the time you get to Saxon Algebra 2 you're doing some long-chain dimensional analysis; more than once I've lost my place and had to start over.

• having the student write two unit multipliers for each conversion (1 yd/3 feet versus 3 feet/1 yd) is a very good thing to do.

• dimensional analysis word problems are also a very good thing to do. For me it was a terrific exercise to use dimensional analysis to solve everyday word problems I had never used DI to solve before.
  

• Terrific DI problem from Saxon Math: The Adams' car has a 16-gallon gas tank. How many tanks of gas will the car use on a 2000-mile trip if the car averages 25 miles per gallon? 
(source: Saxon 8/7 Lesson 96 page 660 #3 - answer: 5 tanks)

  
  

Dimensional analysis is the simplest procedure on the planet, and yet it's strangely challenging to learn. I think this is entirely due to Wickelgren's observation about all math looking alike.

Dimensional analysis is the ultimate exemplar of the practice, practice, practice theory of knowledge.

It's easy, but it's confusing.

Practice solves that problem.

greatest hits: Wickelgren on creativity

from ktm 1

Creativity is an outgrowth of learning, and a lot of it. The past twenty-five years of cognitive psychology research has shown that the more a person knows about a subject, the more creative he or she can be in it. No question an adult poses is considered creative if someone else has already asked it. Thus, an adult must know what has come before to ask creative questions.

This is true more generally as well. A student's ability to be creative in any area of knowledge increases with his or her knowledge of that area. Knowledge forms the fodder for creative new ideas.

This was a revelation to me.

For years I'd been interested in creativity, and had been trying to read books and articles on the subject, none of which told me anything at all.

Finally I gave up.

When I read this passage in Wickelgren, all became clear.

Creativity, like problem-solving and conceptual understanding, is an emergent property.

I had been looking for some kind of essentialist, biological, "trait" explanation of creativity.

The reason I couldn't find it was that creativity develops in the wake of learning.
_______________

Math Coach (the book that started it all for me)

I think we've resorted to drill and kill

My 5th grader just showed me her home work for the weekend. 26 pages, totalling 115 problems. Every single problem involves estimation.

Due Monday.

My daughter was surprisingly upbeat about this. It could have been worse. A friend of hers has 6 of these packets to do over the weekend.

It's panic time. The CMT testing begins in 4 weeks. We have a week off for winter break starting next weekend. Apparently all the fifth graders were tested for their weak areas and some extra practice was assigned.

If you were the parent of the child that came home with close to 600 problems to complete in one weekend, what would you do? Especially if this should come after 5 months of haphazard, math box type homework. This is so completely unreasonable and unproductive.

Why don't they just use one solid curriculum with distributed practice and feedback that builds cumulatively and consistently throughout the year?

I'm all for repetition and practice, but this is nuts. Just before the test, assign 100s of problems to do at home? When the kids get decent CMT scores, are we really going to attribute that to Everyday Math? The answer is sadly, yes.

Needless to say, we are taking a break from Singapore Math this weekend. My plan had been to finish the unit on ratios and review a little of the fraction stuff we did last week. It had been going so well. The ratio chapter was so logically tied into the work we had done on fractions. She was actually enjoying it and it came very easily.

But it is all estimation all weekend instead. This is not time well spent.

Plus, we have problems such as this:

Sara has 5 pet dogs ranging in weight from 65 pounds to 130 pounds. Which could be the number of pounds the dogs weighed in all?
200
400
600
800

Well, 5x65=325 and 5x130=650; Both 400 and 600 should be correct answers as they both fall within the range.

This infuriates me.

math isn't just math

To my last update, where I said:

He just doesn't understand why he should write "Let x equal the number of pears" at the top of the problem. I didn't either when I was his age, but I did it because I had no choice (that's the way math was taught back then). Now, I understand why, and that's why I'm passing it on to him.

Catherine responded:

What is the reason?!

It seems like a good thing to do, but that's all I know.

Because math isn't the only reason to learn math. We benefit at least as much from the sequential, linear, logical thought process, because we can apply it to nearly every facet of our lives, and not just the quantitative ones.

The traditional formalism of math is the embodiment of that process. By llearning it, and being forced to reproduce it every time we do a problem, we learn the process itself, of breaking a problem into its component parts, and creating a step by step solution, where each step follows from the previous steps.

It's discipline for the mind.

This is one of my major objections to "fuzzy" math, that students never learn this logical process.

New York state math standards

2nd exposure

Yesterday Ms. K taught a lesson on dimensional analysis.

The last time she taught a lesson on dimensional analysis was March 10, 2006.

Today is February 9, 2007.

According to the math department an 11-month gap between a first exposure and a second exposure is fine.

It's more than fine, actually.

If a student has been exposed to a topic for one week in 6th grade, and then again for another week in 7th grade, he should be ready and able to take a test.

And not just any test, either. He should be ready and able to take a complicated test filled with multi-step first-applications of the topic or skill.

I'm not surmising this, by the way.

Ed and I were directly told this by the math chair, who was defending Ms. K's latest test which half the kids had hosed, and which we had no interest in discussing in any event no matter how many kids hosed it. We've given up on Ms. K's tests. We've given up on Ms. K! We'd come to discuss curriculum and pedagogy; the math chair had come to defend the test. Under no cricumstances, she said, would she discuss curriculum and pedagogy with a parent. Any parent.

So she discussed the test and we discussed curriculum and pedagogy.

That's how we know the chair of the math department thinks 11 months between exposures is fine and the kids should be ready to take a test.



This is the kind of thing that gets me even more revved than I already am.

I'm handing this one off to Mr. Engelmann:

Typically about 60 school days pass before any topic is revisited. Stated differently, the spiral curriculum is exposure, not teaching. You don't "teach" something and put it back on the shelf for 60 days. It doesn't have a shelf-life of more than a few days. It would be outrageous enough to do that with one topic-- let alone all of them.

...Don't they know that if something is just taught, it will atrophy the fast way if it is not reinforced, kindled, and used? Don't they know that the suggested "revisiting of topics" requires putting stuff that has been recently taught on the shelf where it will shrivel up? Don't they know that the constant "reteaching" and "relearning" of topics that have gone stale from three months of disuse is so inefficient and impratical that it will lead not to "teaching" but to mere exposure? And don't they know that when the "teaching" becomes mere exposure, kids will understandably figure out that they are not expected to learn and that they'll develop adaptive attitudes like, "We're doing this ugly geometry again, but don't worry. It'll soon go away and we won't see it for a long time"?

The Underachieving Curriculum judged the problem with the spiral curriculum is that is lacks both intensity and focus. "Perhaps the greatest irony is that a curricular construct conceived to prevent the postponing of teaching many important subjects on the grounds that they are too difficult has resulted in a treatment of mathematics that has postponed, often indefinitely, the attainment of much substantive content at all."

War Against the Schools' Academic Child Abuse, pp. 108-9

The good news is, I spent the past week having Christopher do dimensional analysis problems.

hah!

I've been teaching Christopher how to use unit multipliers off and on since January 24, 2006. It's been more off than on, extremely sloppy teaching.

But it's been "on" enough that he always has some residual memory when I wake up one day and remember I haven't given him any practice on unit multipliers in a great long while.

I desperately need an afterschooling drill-and-kill book.

I need a book that has pages and pages of dimensional analysis problems of all kinds, along with pages and pages of various multi-step complex problems using algebra, geometry, baby statistics & probability, stem and leaf charts, etc. - I need it all.

In one book.

Anyway, about a week ago I started having Christopher do dimensional analysis problems every day. Five or six of them. My goal this time, and I'm sticking with it until it happens, is for Christopher not only to be able to do dimensional analysis problems, but to do them fast.

We're going to carry on doing dimensional analysis problems until the state test in March; then I'm going to write on my calendar the next date he should do some more of them.

question:

when is that date?

how long am I supposed to wait?

how long can I wait?

I bet Engelmann knows.

One of these days I'll get around to reading his book.

After Christopher gains speed and accuracy I am going to carry on having him do dimensional analysis for the next 2 years so he'll remember unit multipliers for the rest of his life.

[pause]

Oh, fine.

I can no longer find the Dan Willingham article I was positive said that if you study the same thing 3 years in a row you remember it forever.

sigh

The good news is that because I just spent a week having Christopher do dimensional analysis problems he was able to do Ms. K's overly-complicated homework (multi-step dimensional analysis word problems) with ease.

In fact, multi-step dimensional analysis word problems were exactly what he needed.

I hope this is evidence I'm beginning to channel the mind of Ms. K.

Life would be a lot easier around here if that were the case.

rules for installing a new curricula

dropping in for a quick update

Just a quick update. We've finally gotten to Cartesian geometry -- after they did linear and quadratic equations (well, "did" in the current "let's mention it then move on to something else" sense), but only barely. The worksheet had a graph with little cartoon bubble labels: slope, x-axis, y-axis, intercept. The slope bubble pointed to the line, which could just as easily be the equation, but perhaps I'm being picky. Below it was a question: If x is 5, what will y be?

It would have been a perfectly reasonable question except that the tics on the axes weren't labeled. Were they 1, 2, 3, ..., or 2, 4, 6, ..., or 5, 10, 15, ... ? That little glitch made it just a tad difficult to answer the question.

That was presented along with set theory, which was your basic Venn diagrams, with unions and intersections.

Then they went back to "probability and statistics" (and yes, those are sneer quotes). I've already said I think it's bizarre to teach either in the 8th grade, but if you're going to teach it, then teach it. There was no new information presented the second time around. "Probability" was nothing more than your standard ball problem ("If there are 8 green balls and 4 red balls in the hat, what is the probability that you will select a red ball?"), which explains embarrassments like this. Worse was the "statistics" component, which was nothing more than median, mean, and mode.

I hate to break it to the math ed folks, but statistics is not "soft," and it is far more than measures of central tendency. Ultimately, even the hard sciences come down to statistics. Carbon-14 dating (and potassium-argon dating) are statistics. DNA testing is statistics. Epidemiology is statistics.

The math ed people I know wouldn't know a frequentist from a Bayesian, or MANOVA from a t-test. Call me cynical, but I can't help but wonder if that doesn't have something to do with this mess of a curriculum. Why revisit the same concepts over and over again? Couldn't they at least introduce -- in concept, if nothing else -- standard deviations or sample v. population?

If you're going to teach it, teach it. That's my outmoded, stale, dinosaurian view, anyway.

We did the "probability" and "statistics" worksheets in fifteen minutes. That's how much substance there was. But there were terms on the worksheet (she'd copied this one from somewhere, you could tell that) they hadn't covered (from the original source). She had told them to ignore anything they didn't understand (what kind of advice is that for a teacher to give a student?) but he wanted to know what they meant. So we talked about the normal distribution, standard error, and standard deviation (the terms from the original source on the handout).

(By the way, there's an interesting video of Peter Donnelly discussing common statistical errors here, if you're interested.)

In more general terms, I'm teaching Ricky formalism, to set up his problems in sequential, logical steps. He finds it anal retentive, and I'm not drilling him a lot because it frustrates him, but some every time I see him. He just doesn't understand why he should write "Let x equal the number of pears" at the top of the problem. I didn't either when I was his age, but I did it because I had no choice (that's the way math was taught back then). Now, I understand why, and that's why I'm passing it on to him. His biggest problem is that he's pretty good at figuring out how to solve a problem, and he doesn't see why he can't skip steps if he knows the intervening ones. I was like that. But there's a reason for it -- so he won't be like these students.

Anway, back to the beef and noodles.

book club - fear of knowledge

subtitle:
Against Relativism and Constructivism

parentalcation: the bomb or just bombing?

parentalcation: The Bomb or just Bombing?

Why you can't trust schools. They will play with stats, take credit for their students successes, and claim cosmetic changes are curriculum changes.

I think Trailblazers is gone

wow

We had divided duties tonight. The 7th grade transition meeting and a meeting with the new assistant superintendent for curriculum were scheduled for the same night (why?) so Ed and I had to go our separate ways.

Both meetings were a vast improvement over anything we've experienced in this district ever.

It was amazing.

Ed went to the meeting with the assistant superintendent.

He sums up the theme of the meeting as:

I came to Irvington for the excellent schools, and I'm disappointed.

("I'm furious and I voted against the fields because I'm furious" appears to have been a subtext.)

I think Trailblazers is gone.

That's Ed's sense.

Parents in K-5 are beyond enraged. They are openly enraged; they are incensed; they are not mincing words. (I would like to be physically present to witness such a thing once in my life.)

department of corrections: This passage gives the wrong impression. These parents are highly articulate professionals who are accustomed to, as Ed puts it "presenting themselves in public." All of them are capable of containing emotion while expressing strong views.

This is a skill I lack. I've been sitting alone in a room writing for so long that I simply have not developed an ability to speak passionately, persuasively, and extemporaneously in public.

These are parents I've never met & may not even know by face.

The curriculum advisor said the math wars are like the reading wars; they've just about run their course.

"New textbooks will be coming out."

That's what she said. (paraphrasing)

New textbooks will be coming out.


next up: balanced math!

New textbooks coming out is fine.

Actually, it's great.

But the fact is, we lost the reading wars, didn't we?

We're all sitting around thinking we won; we're thinking common sense and peer-reviewed science prevailed.

They didn't.

Whole language was repackaged as balanced literacy, and the ed schools and their associated NGOs went on their merry way.

The reports I have from parents at Dows Lane are that we are using balanced literacy; we have hired a Dows Lane administrator, educated at Columbia, who has a "specialty" in balanced literacy; we have high rates of dyslexia; we have numerous "reading specialists" on staff; and we are refusing to pay for Windward School although we are apparently recommending to parents that they spend the $40,000/year it takes to send their kids to the school.

We can't teach them to read for $20,000/year. No, that takes $40,000.

This is what I'm told.

So. If the math wars are going to run their course the same way the reading wars ran their course, that's a problem.

I've decided to get out in front of the curve for once in my life and declare myself against balanced math.

There's no reason to wait for new textbooks that will be coming out.

Let's adopt one, two, or all three of the excellent textbooks we have on the market now.


speaking of losing the reading wars...

Some kind of Tri-State Consortium report was presented at the Board meeting, and it's Lucy Calkins all the way.

Irvington is to be Lucy Calkinsized, it seems.

Lucy Calkins.

Godmother of balanced literacy, Lucy Calkins.

It's in the works.


That is a very bad idea.


more tomorrow

The 7th grade transition meeting was great.

Amazing.

With caveats.


what are the excellent texts on the market now?

from NYC HOLD:

The California Department of Education has a careful content-based adoption process for K-8 curricula. Reports may be found through the CDE site for Mathematics Frameworks and Curricular Materials. David Klein at CSU Northridge also has links to Content Review Panel reports on middle school mathematics programs. The official final adoption report of 2001 provides positive reviews of K-8 materials that include for grade school Saxon Math K-6, Sadlier Progress in Mathematics CA Edition K-6, Harcourt Math CA Edition 2002 K-6, Houghton Mifflin Mathematics CA Edition K-5, and Scott Foresman CA Mathematics K-6; for middle school the adopted programs include McDougal Littell Structure and Method (the venerable Dolciani series), and Prentice Hall Pre-Algebra and Algebra 1.

In addition to the named programs adopted in California the Singapore Primary Mathematics K-6 curriculum is uniformly recommended by subject matter experts, and also the Singapore New Elementary Mathematics grades 7-10 series is of high quality.

link for GramSchmidt Identity

GramSchmidt Identity

to read

I'm not going to have get to this any time soon, so I'm hoping someone here has the time to take a look:

An Experiment in Teaching Ratio and Proportion (pdf file)

Here's the abstract

This article appears in the journal Educational Studies in Mathematics, which may have most of its articles posted online. Not sure.


Bishop & Hook on Saxon Math in California (pdf file)

Bishop & Hook's paper is here

abstract

another video

vocabulary word for the day

thenar eminence



I'm reading Ian Osborn's Tormenting Thoughts and Secret Rituals, which I think Karen told me about.

In med school Osborn suffered from an obsession involving his thenar eminence:

I would have the sudden, intrusive image of me standing at a patient's bedside ready to draw a sample of blood: I unsheath a large-bore phlebotomy needle, menacing, daggerlike in its appearance, and then inexplicably, instead of inserting the needle into my patient's vein, I thrust it to the hilt into the thenar eminence of my hand.

omg

back to work

Steve H on balanced literacy

This means phonics as a last resort in fourth grade. That's what it means at our schools.

a theoretical mathematician evaluates constructivist math

This statement, from a theoretical mathematician, was first posted to the Bridgewater-Raritan Parents Math Forum.

I am a theoretical mathematician (Ph.D. UCLA 1996), who taught as an Adjunct Assistant Professor at UCLA for 2 years ('96-'98), and then as an Assistant Professor at Illinois State University (ISU) for 4 more years ('98-'02). In addition to my experience teaching college math and computer science, through interaction with many of my colleagues at ISU I became well-versed in issues of Mathematics Education. (ISU has one of the largest Math Ed. programs in the country.) In fact, many of my fellow faculty were involved in drafting the NCTM standards, both past and present.

Both my daughter (eighth grade) and my son (fourth grade) have used EDM exclusively for their in-school math instruction. As a mathematician I find the program abysmal, and I know that I am not alone (amongst mathematicians and others) in this assessment.

Let me share with you a portion of an email that I sent to our local (Hollis, NH) school board. This should serve to encapsulate (at least in part) my position on EDM.

As you could tell, I am passionately opposed to the use of Everyday Math (EDM). My experience with it, both personal and professional, has been uniformly negative. I also have large amounts of anecdotal evidence that confirms that the only way our kids learn any math while using EDM in school is when parents become frustrated and just teach them math the "old fashioned" way.

What I object to is the "Emperor's New Clothes" syndrome: everybody telling me how great this program is, but there being absolutely no evidence that it provides any benefit at all. What is particularly telling are the words and phrases that its advocates use: "It makes math more enjoyable," or "The kids really like the games." Of course they do!

What I believe has happened is that the teachers have been sold a bill of goods: most elementary and middle school teachers, while being dedicated and tireless in their devotion to wanting to teach our children, have not received adequate training in mathematics. (This I can attest to from first-hand experience; I once taught Calc I to a group of students destined to be "math teachers." I failed half of them (many couldn't do high school algebra). What was particularly disturbing was the fact that failing my class did not dissuade them from wanting to be teachers, it merely "redirected" them: without passing Calculus they simply could no longer be Secondary (i.e., High School) math teachers; Calculus, it seems, wasn't required for Elementary or Middle School (math) teachers.)

Hence, when a program (endorsed by "experts") comes along and tells them that they can do a better job teaching math by having the kids participate in group activities, making it "relevant" to their "everyday" lives, the teachers rush to adopt it: who wouldn't? However, the hard yet honest fact is that math is difficult, and requires work, dedication and perseverance to master. As Euclid said, "There is no royal road to mathematics."

But beyond all this, what troubles me most is the fundamental philosophical flaw in EDM: It ignores the core beauty and power of mathematics, viz., that it is an edifice constructed out of pure reason, all of whose inferences and deductions flow logically and unarguably from more basic facts. EDM asks the students to flit willy-nilly from room to room or even floor to floor in this structure, without ever exposing them to the skeleton, the underlying architecture.

The basic premise of EDM, so much so that its part of its name, that math should be valued or appreciated only insofar as it can be applied to "everyday things," is worse than misguided, it is a lie promulgated by people who, quite frankly, don't understand the first thing about mathematics. (Example: Do we study "Everyday English Literature?" Why do we still read Shakespeare? Are people really worried about being encountered by three old women stirring a big pot, and wanting to know how to deal with them?)

Let me recount for you what I used to tell all my students the first day of class: Being in a (math) class is like buying a membership to Gold's Gym. If you come to class, sit passively by, and then complain that you didn't learn anything, that you just don't "get it," that is akin to walking into the gym a month after you bought your membership and complaining that you haven't gotten any stronger, even though you come to the gym everyday and watch people work out. Being in a class, or in school, provides only the opportunity to learn, the teacher is there to facilitate the learning process, but the effort must emanate from the student.

In short, the "guided instruction" methodology, however well-intentioned, is in fact, "misguided": Imagine paying a tennis or golf pro to help improve your game, only to have her tell you to "try and discover the right method to strike the ball on your own." You would be justifiably outraged; you pay someone who is a better tennis player / golfer than you to teach you the right way to do it. Human minds are not designed to do math (unlike, say, to learn language); they need to be taught the right way to do it."

I hope that it comes through in what I have written that I am not blaming the teachers. In my (admittedly limited) experience, many of them are similarly frustrated by having to adhere to an administration-mandated (math) curriculum that they neither support nor believe in. I have rarely met a teacher who is not extraordinarily dedicated to her students, and I absolutely do not want anything I say to be construed as being critical of the job that they do. My point here was merely to demonstrate that some teachers (as well as administrators, school board members, etc.) are insufficiently trained to evaluate properly the merit of their math curriculum, and so rely upon others (like the NCTM) to do so for them.

Tony Falcone, Ph.D

This passage is beautiful:

The basic premise of EDM, so much so that its part of its name, that math should be valued or appreciated only insofar as it can be applied to "everyday things," is worse than misguided, it is a lie promulgated by people who, quite frankly, don't understand the first thing about mathematics. (Example: Do we study "Everyday English Literature?" Why do we still read Shakespeare? Are people really worried about being encountered by three old women stirring a big pot, and wanting to know how to deal with them?)

The night before Tony's statement appeared on the B-R Mathforum I had been trying to explain to Ed why constructivist math, from the perspective of a real mathematician, isn't even math.

Of course, I can't really explain it, not off the cuff at any rate.

Then Tony's statement landed in my email queue.

We are all lucky to have it.

New video from "Where's the Math?" folks

The new video from the Where's the Math?" folks is located here



Very well done.

report: Reading First has worked

I had been hearing that Reading First had worked, mainly in interviews with E.D. Hirsch, I think:

And that, paradoxically, is the one area which has just come in for a scandal, the reading first program, because that program insisted that you have to have a phonics-oriented early reading program which was just what they have been pilloried for in this recent GAO report.

I'd been asking Ken about it, because I figured if anyone would know, he would.

Ken said he'd heard the same thing, but didn't know.

Turns out Hirsch was right.

Reading First has worked; Ken reports that it is one of only four programs in the Department of Education - and the only program within NCLB - rated "effective."


Reading First

• Reading First is a focused nationwide effort to enable all students to become successful early readers.
• Funds are dedicated to help states and local school districts eliminate the reading deficit by establishing high-quality, comprehensive reading instruction in kindergarten through grade 3.
• Building on a solid foundation of research, the program is designed to select, implement, and provide professional development for teachers using scientifically based reading programs, and to ensure accountability through ongoing, valid and reliable screening, diagnostic, and classroom-based assessment.

4 of 88 programs rated "Effective "

By my count, 4 of 88 Department of Education programs are rated "Effective":

• Adult State Education Grants "The Adult Education State grants program funds literacy and basic skills education programs to help adults become literate, get a secondary school education, or learn English. Funds are distrbuted by formula grants to States and States must distibute funds competitively to local providers." (that's good news)
  

• NAEP ("The Nation's Report Card")
  

• NCES (National Center for Education Statistics)

• Reading First

whole language lives on

New report out from Fordham; schools still disguising whole language as balanced literacy:

Moats, a psychologist and widely respected authority on early reading, authored a previous Fordham report in October 2000 called Whole Language Lives On. In it, she uncovered many whole-language programs hiding behind the phrase "balanced literacy" in order to win contracts from school districts and avoid public scrutiny.

Seven years later, such programs still exist-and still try to pull the wool over educators' eyes. Worse, major school systems, including Denver, Salt Lake City, and New York City, continue to adopt them, misled by materials that "talk the talk," touting the five elements of effective reading instruction identified by the National Reading Panel, but which are actually just whole-language programs in disguise.

Here's Mike Petrilli:

"This report's findings help to explain why the federal government has to be prescriptive in its implementation of Reading First," said Michael J. Petrilli, Fordham's Vice President for National Programs and Policy. "Anyone can put the label ‘scientifically-based' on the cover of their reading program. But if we want to do right by kids, we need to dig below the surface. If the policy is to fund only programs that truly work, officials at all levels need to fend off the charlatans."

This is where I team up with Engelmann.

It's time for think tanks, policy wonks, and researchers to stop telling us about the "five principles" of effective reading instruction or whatever it is, and start telling us the names and authors of the programs that work.

But no.

Instead, Moats tells parents to ask their school, Does our school reading program--

• Have valid screening measures in place to identify children at risk and provide them with early/extra instruction in word recognition, comprehension, and writing skills?
• Interweave multiple language components (such as speech sounds, word structure, word meaning, and sentence structure) together in the same lesson?
• Support reading comprehension by focusing on a deep understanding of topics and themes rather than developing a set of shortcut strategies?

So say you ask your school these probing questions.

What's the answer going to be?

NO?

We DON'T interweave multiple language components such as speech sounds, word structure, word meaning, and sentence strugure together in the same lesson?

School district personnel, in my experience, routinely tell parents programs are "scientific," "supported by research" and all the rest whether they are or not.

My favorite instance of this was the time our former middle school principal told a huge gathering of parents that "all the research shows constructivist math is the way to go."

The only way to make that a true statement is to change "all" to "none."

He made this remark with aplomb.

Parents don't have a chance against aplomb.

My district uses balanced literacy, I'm told.

In case you were wondering.



Advanced Placement Test Fees and AP Incentives rated moderately effective

These are programs designed to increase minority participation in AP courses.

By my count 7 programs, of 88, are rated "Moderately Effective."

Not bad.


Reading First has worked
Reading Last

dimensional analysis word problem

I love this guy.

Because you never learned dimensional analysis, you have been working at a fast food restaurant for the past 35 years wrapping hamburgers. Each hour you wrap 184 hamburgers. You work 8 hours per day. You work 5 days a week. You get paid every 2 weeks with a salary of $840.34. How many hamburgers will you have to wrap to make your first one million dollars? [You are in a closed loop again. If you can solve the problem, you will have learned dimensional analysis and you can get a better job. But, since you won't be working there any longer, your solution will be wrong. If you can't solve the problem, you can continue working which means the problem is solvable, but you can't solve it. We have decided to overlook this impasse and allow you to solve the problem as if you had continued to wrap hamburgers.]

He's got one about college applications, too:

A Wilton High School senior was applying to college and wondered how many applications she needed to send. Her counselor explained that with the excellent grade she received in chemistry she would probably be accepted to one school out of every three to which she applied. [3 applications = 1 acceptance] She immediately realized that for each application she would have to write 3 essays, [1 application = 3 essays] and each essay would require 2 hours work [1 essay = 2 hours]. Of course writing essays is no simple matter. For each hour of serious essay writing, she would need to expend 500 calories [1 hour = 500 calories] which she could derive from her mother's apple pies [1 pie = 1000 calories]. How many times would she have to clean her room in order to gain acceptance to 10 colleges? Hopefully you didn't skip problem No 1. I'll help you get started.... 10 acceptances [ ] [ ] etc.

brain scans will save the world

See?

It's all biological!




Sorry, I couldn't resist. This image is just too funny. Takes me back to my NAAR days (Nat'l Alliance for Autism Research) when scientists on our SAB would sit around heaping scorn on brain scans and what journalists & regular people thought they were learning from them.

The article is actually pretty interesting. Also, I have the sense that brain scans have come a ways since I was vetting research proposals, though perhaps one can't say the same for the field science illustration.

Fast language learners boast more white matter
New Scientist

middle school model

The administration appears determined to implement the middle school model here in Irvington. Topic is up for discussion at the Board meeting tomorrow night. I imagine it's a done deal.

Apprised of these facts, Ed said, "We should go to that meeting."

No way.

Let someone else go to that meeting.

We have one more year in this school -- well, one and a half -- then it's so long and farewell!

This is my feeling.

I must say, the new principal does have some political savvy. I like the guy, and I don't particularly feel like attending the Board meeting in order lob hand grenades at the middle school model.

So I've contented myself with lobbing one great big, pulsing hand grenade via the Irvington Parents Forum.

That one fell into my lap. A friend emailed asking whether I knew anything about the middle school model.

I did.

What I didn't know was that the NMSA website, the officially cited organization that is to middle schools what the NCTM is to math, contains pages and pages of Public Relations Resources for "middle level educators."

Nor did I know that the NMSA is of the opinion that:

There may be no task more important today for middle level educators than public relations. National Middle School Association (NMSA) is committed to helping you build support for quality middle level schools. Join us in taking action to gain visibility for successful middle grades programs and practices by using the following resources designed to help you be a successful public relations practitioner.

Oh yeah, that's the hard sell.

My line on public relations for educators is:

Any organization or corporation that has to develop marketing campaigns to convince parents of its worth is an organization or corporation selling something I don’t want to buy.



Quick PR Ideas

"practical, low-cost, proven public relations ideas that have worked in schools everywhere"

such as -

Have Parents Speak Up for You

Encourage your PTA or PTSA to sponsor a "Get to Know X Middle School—It's a Great Place to Learn" night at feeder elementary schools. Invite the elementary parents to the meeting and have your parents explain the value your school provides students. Encourage questions. Your parents can deliver a highly credible message to other parents.

or:

Let Students Demonstrate Their Computer Skills

Create a program where your students teach senior citizens computing skills. Email can help seniors keep in touch with their family and the world, but many of them aren't confident using computers. Your students can teach them the basic skills. Students might also play computer games with seniors and write them occasionally. Let seniors know that the school can serve them.

or:

Use Themes

Themes can communicate what people can expect from your school. If you don't have a meaningful theme, get one. Then look for every possible place to use it-school newsletters, home page of a school web site, lunch menus, report cards, calendars, school letterhead, and even envelops since more people see the envelop than the letter.

yup

That ought to do it.

You've got parents so ticked off about curiculum, pedagogy, and student achievement that they're voting down bonds, walking out of math nights, and writing blogs - TIME TO CREATE A THEME!

TIME TO CREATE A THEME AND USE IT EVERYWHERE INCLUDING REPORT CARDS, SCHOOL CALENDARS, AND EVEN ENVELOPES BECAUSE MORE PEOPLE SEE THE ENVELOPE THAN THE LETTER!



how to write an op-ed

What Is an Op-Ed Article?

An Op-Ed or Opinion Article is an opinion piece published in a newspaper but written by someone who is not on that newspaper's staff. Many large dailies, smaller dailies, and weekly newspapers use op-eds somewhere in their editorial section. On many large newspapers, that paper's editorials, the editorial cartoon and columns by staff writers will appear on one editorial page. Opposite that page, the op-ed articles will be run, and that's where the term "op-ed" comes from—it's opposite the editorial page.

The important point is that these articles provide anyone with the chance to publish his or her opinion. You don't have to convince a reporter to cover something; you can express your opinion. You may see that the president of the chamber of commerce is published in the op-ed columns. This opportunity is available to you, too.

question: Do middle level educators not know what an op-ed article is?

This stuff must be mortifying to actual middle school teachers.


how to write an op-ed article, part 2

So What Do I Do?

First, determine whether newspapers in your area use op-ed articles. You can do this simply by reading the editorial pages. See if national columnists or local officials are published. Read these articles. Become familiar with style, length, format, messages, and anything else that makes them stand out.

Second, decide what you would like to write. Sample topics for educators might include:

• How parents can help students learn
• What's right with education
• Success of our local school
• The importance of middle level education
• The need for resources in education
• How the community can support its school

I'm starting to grasp the obvious reality that these organizations are even more condescending to teachers than to parents.

Or not.

The sample op-ed article, provided to middle level educators for their use, is a 666 word essay (666!) advising parents on ways to ways to help young adolescents grow.


theme contest

So say you were a middle level educator trying to drum up enthusiasm for the middle school model.

What theme would you pick?

Entries should be dated no later than midnight February 28, 2007; no limit on number submitted per household.

The Outrage of Project Follow Through: - Chapter 3

http://zigsite.com/PDFs/FOLLOW_THROUGH/FT-Chap3.pdf

Chapter 3 is out... yeah! I am not going to bother analyzing it, because others are much more adept at that than I am, but I do want to say one thing. This chapter literally made me shake with anger.

One of the recurring themes in the book, was how much the school establishment worked against the implementation of the Direct Instruction model. I found myself cussing the various antagonists out under my breath as I was reading. The stupidity of some of the characters is amazing.

There was one bright spot in the chapter though. In a fairly long and very descriptive passage, Zig describes a well run kindergarten class room. Here is a teaser:

As soon as the bell rings, the teacher says, “Everybody, you can finish your worksheet later. It’s time for our morning warm-up. So get those thinking engines ready to go. The blue group is ready ... so is the yellow group.”

The aides are positioned on each side of the room. The teacher walks to the chalkboard. “We’re going to start with the days of the week. Tell me what day it is today ... Get ready.”

The teacher claps. As she does, nearly all of the children respond, “Tuesday.”

The story is much more involved, but illustrates how the teacher and the aids work together, like a well oiled machine. It's amazing how much attention to detail is given to every aspect of the learning environment.

As I read the passage, I couldn't help to get a little depressed as I thought about all the trouble my bright 1st grader is having reading, yet here is a story about poor kindergartners from disadvantaged homes who were performing above the level my daughter is now. It's enough to piss you off.

(cross posted at parentalcation)

help desk

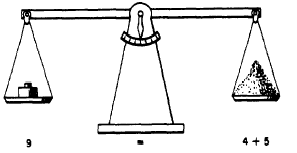
Ed and I are plotting strategy for the state math test & for the rest of the school year.

I need advice.

Sometime this weekend it came to us that we have a need for speed.

speed and accuracy: the KUMON motto

Christopher managed to move up from a D- on his next to last test (a highly inflated D- may I add) to a genuine C on the last test by dint of mighty reteaching and home-assigned distributed practice.

He needs to move to a B.

He also needs to return to a 4 on the state test this year.

Since we're not going to be able to achieve this through our preferred medium of sound curriculum and pedagogy, we're going to have to find a work-around, and that work-around is going to be speed.

He has to practice until he's fast. That way he can whiz through the problems he does know and have a shot at cracking some of the problems he doesn't know. (Meanwhile my friend Kris says she's begun to think like Ms. K; she's managing to figure out what will be on the test that the kids have never seen before. Kris took calculus in college, so she can do these things. Next test, she's going to brief me.)

Anyway, speed.

Here's the question.

These days kids are taught to "do the same thing to both sides" of an equation vertically instead of horizontally.

I can't find an image of it online, and I can't get the spacing to work, so you'll have to imagine it from this:

3x+2 = 5+2x
- 2x = - 2x
x + 2 = 5 + 0

Then you do another vertical subtraction, writing a below the 2 on the left side and the 5 on the right side.

I like this procedure because it makes obvious the fact that you're subtracting (or adding) when you "do the same thing to both sides."

But it takes forever, and it eats up space since Christopher's handwriting is still big and not neat. (Sure glad the schools don't bother teaching handwriting to mastery any more! Why would we waste time teaching legible handwriting when we've got keyboards??)

I'm going to teach him how to solve equations horizontally and have him practice until he can do it fast.

But how should I do this?

Russian Math shows students what happens when you add or subtract the same quantity from both sides: you change the sign.

From that point on the book has you simply move variables and constants from one side to the other, changing the sign as you move:

3x + 2 = 5 + 2x
2 = 5 + 2x - 3x

That's the fastest method, obviously.

Also the most space-saving when you have enormous handwriting.

But Ed says doing things that way gives you more likelihood of error, and I should have Christopher write out what he's doing:

3x + 2 = 5 + 2x
3x - 3x + 2 = 5 + 2x - 3x

etc.

Any thoughts?



We are also going to drill dimensional analysis until Christopher can do it in his sleep.

_________________

image source:
Linear Equations in One Variable

throwing money at the problem

OK, so I went through our upcoming State Test and pulled out a rough version of topics to be tested.

Then I went through my five gazillion workbooks looking for suitable worksheets.

Results?

I don’t have any worksheets on Venn diagrams.

Or on circle graphs.

Or on problems like “The diameter of a circle is X; find the radius.”

Or on counting and permutations.

Also, I don't remember what counting and permutations are.

So I’ve just ordered ... oh, maybe another hundred fifty bucks worth of workbooks that will eat up the remaining free space on my office floor and possibly make the difference between a 3 and a 4 on the state test not to mention that between amnesia and something akin to mastery on the critical skill of constructing a circle graph.

I don't know why I'm doing this.

After all, the slim little $11 practice workbook the school just made us all buy ought to be plenty.

Say you're a 7th grader who's constructed one circle graph in your life, and that was last year.

What do you need to do this year to be prepared to construct a circle graph on the state test?

Construct another one!

Just one!

In your slim little $11 test prep booklet!

One circle graph last year, one circle graph this year.

That'll do it.

____________

see also:

to do
state test coming right up (2006)
throwing money at the problem
more stuff only teachers can buy
help desk 1
state test coming right up (2007)
help desk 2
my life and welcome to it
inflammatory
canadianteacher.com
progress report
despair
28 out of 30