Posts
I've been cruising The Corruption of the Curriculum [Fordham Foundation link] for awhile now.
Just snapped up a copy for five bucks at Amazon.
Saturday, July 21, 2007
glutton for punishment
I've been cruising The Corruption of the Curriculum [Fordham Foundation link] for awhile now.
Just snapped up a copy for five bucks at Amazon.
teach to the middle redux
"We were told to cross off the kids who would never pass. We were told to cross off the kids who, if we handed them the test tomorrow, they would pass. And then the kids who were left over, those were the kids we were supposed to focus on."
source:
Is NCLB Leaving Children Behind?
via: Fordham Foundation
It's always worse than you think.
(for newbies, I'm pro-NCLB, with the occasional bout of exasperation.)
speed test
The precision teaching folks distinguish amongst:
After discovering that C. can solve only 50 simple addition problems in 60 seconds when he should be able to do between 70 and 90, I decided to find out how fast he can write digits.
I had him write the digits 0 - 9 over and over again as fast as he could for 60 seconds.
He wrote 110, 10 more than the performance standard the PT folks seem to use.
So we're going to be doing Saxon Fast Facts sheets, Books 7/6 and 8/7 (the Tests and Worksheets Bookets), until he's up to speed.
Literally.
I'm also starting both of us on Cursive Writing Skills from Megawords. His handwriting is horrific, as is his printing, and it's not getting better over time.
back to basics
speed test
- tool skills - how many digits can a student read or write per minute
- component skills - how many simple addition problems student can solve per minute
- composite skills
After discovering that C. can solve only 50 simple addition problems in 60 seconds when he should be able to do between 70 and 90, I decided to find out how fast he can write digits.
I had him write the digits 0 - 9 over and over again as fast as he could for 60 seconds.
He wrote 110, 10 more than the performance standard the PT folks seem to use.
So we're going to be doing Saxon Fast Facts sheets, Books 7/6 and 8/7 (the Tests and Worksheets Bookets), until he's up to speed.
Literally.
I'm also starting both of us on Cursive Writing Skills from Megawords. His handwriting is horrific, as is his printing, and it's not getting better over time.
back to basics
speed test
sling the lingo
Vicky S left this link awhile back:
educational jargon generator
I hope they've got the words learner, reflect, and authentic on that list.
educational jargon generator
I hope they've got the words learner, reflect, and authentic on that list.
what is a mile?
from Doug S:
Every once in awhile I'm tempted to reflect (another perfectly fine English language word ruined by edu-texts) upon the fact that I have spent the last 2 1/2 years hanging out with people who know what a nautical mile is and whether it's longer or shorter than a regular mile and by how much.
Doesn't this imply that somebody took a wrong turn somewhere back in the mists of time?
Like, say, in my high school math courses?
So every once in awhile I'm tempted to make meaning out of all this.
Then the feeling passes.
1 mile = 5280 feet = 1760 yards = 8 furlongs = 320 rods.
Unless it's a nautical mile, which is 1852 meters (precisely), 6076.115 ft, or quite close to 1 minute of longitude at the equator (its original definition).
Every once in awhile I'm tempted to reflect (another perfectly fine English language word ruined by edu-texts) upon the fact that I have spent the last 2 1/2 years hanging out with people who know what a nautical mile is and whether it's longer or shorter than a regular mile and by how much.
Doesn't this imply that somebody took a wrong turn somewhere back in the mists of time?
Like, say, in my high school math courses?
So every once in awhile I'm tempted to make meaning out of all this.
Then the feeling passes.
Friday, July 20, 2007
hyperspecificity redux
from Robert Slavin's Educational Psychology:
That's about the speed of things around here.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
birthday and a vacation
* Lave, J. Cognition in Practice Boston: Cambridge Press
The most important thing to know about transfer of learning is that it cannot be assumed (Mayer & Wittrock, 1996). Just because a student has mastered a skill or concept in one setting or circumstance, there is no guarantee whatsoever that the student will be able to apply this skill or concept to a new setting, even if the setting seems (at least to the teacher) to be very similar (Mayer & Wittrock, 1996)….Lave (1988)* describes a man in a weight-loss program who was faced with the problem of measuring out a serving of cottage cheese that was three-quarters of the usual two-thirds cup allowance. The man, who had passed college calculus, measured out two-thirds of a cup of cottage cheese, dumped it out in a circle on a cutting board, marked a cross on it, and scooped away one quadrant. It never occurred to him to multiply 2/3 x 3/4 = 1/2, an operation that almost any sixth-grader could do on paper (but few could apply in a practical situation).
p. 229
That's about the speed of things around here.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
birthday and a vacation
* Lave, J. Cognition in Practice Boston: Cambridge Press
from Jenny D: principal of an elementary school in an affluent town
I was told once by the principal of an elementary school in a very affluent town: "There's nothing we're doing at our school that can top what these kids get at home in their background. We could not teach them a thing in third grade, and at the end of year they'd still score above grade level."
I feel as if Jenny D has just handed me the smoking gun!
affluent schools - poor schools
Today, schools are rated poorly if their students do not score well on state-mandated tests, regardless of whether children’s learning has been helped or hindered by the school environment. By the same token, schools serving affluent families in a resource-rich community are assumed to be good schools on the basis of children’s higher test scores, which may be high even in the face of a mediocre education. Downey and his colleagues [17, 34] have developed a new approach to measuring school performance that accounts for seasonal differences in learning, wherein the portion of student performance that can be attributed to the school is separated from the portion due to nonschool learning periods, including both during the period before a child enters school and over the summers as they progress through school. Using data from the Early Childhood Longitudinal Study (ECLS), they find striking differences in school impact with this approach:
[O]ur analyses of reading suggest that 70 percent of currently labeled “failing” schools are not really failing…Many teachers and administrators working in schools serving disadvantaged children face a variety of challenges including scarce resources, large classes, and little parent involvement. Despite these conditions, a surprising number of professionals serving disadvantaged students appear to be doing a good job, much better than previously thought.
[17 p. 24]
Using this measure of “school impact,” in recent analyses of data from the Early Childhood Longitudinal Study (ECLS), the researchers find that many schools considered “failing”—due to the low test performance of their students—are actually doing a better job of education than schools with much-higher-performing students.
from The Learning Season: (pdf file)
Rorschach blot
from The Learning Season: (pdf file)
Of course there are two ways of looking at this, aren't there?
"Even struggling schools provide some support for children's learning".... or struggling schools don't "provide" much "support" for children's learning and there's not too much learning going on anywhere.
It's pretty horrifying that even policy reports on the subject of the achievement gap are now talking about "support" for children's learning.
We seem to have an entire K-12 philosophy of public education built upon the concept of incidental learning. Kids just naturally learn stuff, the same way they just naturally grow taller every year; the school's job is to "support" them while they learn (and grow).
Which is one of the reasons I particularly dislike the expression "academic growth."
note: the race gap in scores is different from the income gap
The phenomenon of summer undoing school-year learning has come to be known as
“summer learning loss.” It was first commented on in 1906 [29], followed some decades later by the 1978 book Summer Learning and the Effects of Schooling, by Barbara Heyns, which was based on her study of New Jersey students. More recently, a number of researchers [17, 30–37] have found that nearly all the differences in achievement between poor and middleclass children can be attributed to changes in learning that take place over class the summer. This finding is particularly surprising—and important—given that the vast majority of public and philanthropic resources are dedicated to school-year education, and that relatively scant resources are earmarked for summer programs.
While summer learning loss has operated mostly “under the radar,” the effects of early childhood experiences on racial, ethnic, and class test-score achievement gaps have received a great deal of media and research attention. Evidence from a set of longitudinal studies demonstrating that preschool children benefit significantly—and permanently—from early learning experiences [10–12], along with new understandings from neuroscience [4, 38], has formed the foundation for a national movement: public preschool is fast becoming a norm across the country, and public funding for early-childhood care and education is growing.
Despite these growing gaps, research on seasonal learning shows that children in all socioeconomic groups are actually progressing at the same rate during the school year. Yet during the summer middle-class children generally continue to learn, or hold steady, especially in reading, while poor children lose knowledge and skills [42]. These findings are especially surprising, given the well-documented disparities in facilities, teacher quality, curriculum, safety, and materials between schools serving poor children and those in affluent communities [9, 43–46]. Research on seasonal learning demonstrates that even struggling schools provide some support for children’s learning, at least compared with a summer devoid of educational experiences [34, 36, 47].
Of course there are two ways of looking at this, aren't there?
"Even struggling schools provide some support for children's learning".... or struggling schools don't "provide" much "support" for children's learning and there's not too much learning going on anywhere.
It's pretty horrifying that even policy reports on the subject of the achievement gap are now talking about "support" for children's learning.
We seem to have an entire K-12 philosophy of public education built upon the concept of incidental learning. Kids just naturally learn stuff, the same way they just naturally grow taller every year; the school's job is to "support" them while they learn (and grow).
Which is one of the reasons I particularly dislike the expression "academic growth."
note: the race gap in scores is different from the income gap
new summer learning loss study - the achievement gap explained? (and Summercamp!)
Fordham posts a link to a new study & report arguing that much of the achievement gap can be attributed to summer learning loss in poor kids:
We talked about "summer regression" at the old site (summer brain drain, summer reading question, time costs of spiralling curricula)
Reading Fordham's summary of the new research, I feel I'm watching a re-run. Maybe that's unfair, but isn't this the usual correlation equals cause-type reasoning that leads to busing and 5 gazillion initiatives to increase parental involvement in the schools?
Or am I missing something?
It seems to me that the common theme running throughout these studies is that middle class parents are doing a very large amount of incidental and not-so-incidental teaching of their kids - and that schools are failing to "disaggregate the data" concerning the parent contribution.
I think I've mentioned that this was a bit of a moment when we met with the new assistant superintendent who, as I've also mentioned, intends to use classroom and test data to drive instructional decisionmaking. ["drive instructional decisionmaking"?? I may be reading slightly too much Ed Week....]
I like most-to-all of her ideas, but she has a blind spot on the subject of tutoring. She herself hired a math tutor for her son when he was in high school, and she sees this as normal.
Of course, hiring math tutors - hiring tutors of all kinds in all subjects - has certainly become normal. It's the new normal. But that's the problem.
Even if you decide that you're going to have a public school system in which parents do a great deal of preteaching, reteaching, and tutor-hiring, you need to know what parents are doing if you're going to have data-driven decisionmaking.
Data is useless if you leave out major variables.
If an English teacher sends home a writing assignment that's over the students' heads, but a mostly-OK set of papers results because parents have walked their kids through the composition step by step, teachers aren't getting correct feedback on the assignment.
The data is going to tell you the assignment worked, when what worked was parents breaking the assignment down into component parts and teaching each part separately.
..................................
Beyond this, why am I reading about summer camp?
Are these researchers suggesting that middle class kids routinely gain 47 points on reading tests at camp?
(How much is 47 points, anyway? How many points did kids gain during the school year? I may have to see if Ed can get a copy of the article...)
If summer camps run by distracted teenagers are producing major gains in reading, maybe we need some studies of the super-effective teaching methods known only to 18 year old, untrained college kids.
..................................
Speaking of camp, C. and I played hooky Wednesday (though, as C. pointed out, you can't really play hooky from camp) and went to the city to see Summercamp!
You must see it.
Summercamp! is a laugh-cry-embrace-life sort of movie; I don't think I've cried so much in a movie theater since seeing Forrest Gump two weeks after giving birth to twins. [for newbies: my oldest son Jimmy, who was then age 7, is autistic]
An incredible movie - beautiful. There's no crying 'til the very end, and even then it's good crying. I promise.
from the NY Sun review:
Pure pleasure.
Miller presents past research showing that, during the school year, low- and high-SES students make similar progress on standardized tests. Between spring and fall, however, the scores of low-SES students either level off or decline, while those of high-SES students continue to rise. Research by Alexander and colleagues confirms this trend. Tracking 325 Baltimore students, they found that high-SES students gained a cumulative 47 points on reading test scores during the summer, while their low-SES counterparts lost 2 points.
We talked about "summer regression" at the old site (summer brain drain, summer reading question, time costs of spiralling curricula)
Reading Fordham's summary of the new research, I feel I'm watching a re-run. Maybe that's unfair, but isn't this the usual correlation equals cause-type reasoning that leads to busing and 5 gazillion initiatives to increase parental involvement in the schools?
Or am I missing something?
It seems to me that the common theme running throughout these studies is that middle class parents are doing a very large amount of incidental and not-so-incidental teaching of their kids - and that schools are failing to "disaggregate the data" concerning the parent contribution.
I think I've mentioned that this was a bit of a moment when we met with the new assistant superintendent who, as I've also mentioned, intends to use classroom and test data to drive instructional decisionmaking. ["drive instructional decisionmaking"?? I may be reading slightly too much Ed Week....]
I like most-to-all of her ideas, but she has a blind spot on the subject of tutoring. She herself hired a math tutor for her son when he was in high school, and she sees this as normal.
Of course, hiring math tutors - hiring tutors of all kinds in all subjects - has certainly become normal. It's the new normal. But that's the problem.
Even if you decide that you're going to have a public school system in which parents do a great deal of preteaching, reteaching, and tutor-hiring, you need to know what parents are doing if you're going to have data-driven decisionmaking.
Data is useless if you leave out major variables.
If an English teacher sends home a writing assignment that's over the students' heads, but a mostly-OK set of papers results because parents have walked their kids through the composition step by step, teachers aren't getting correct feedback on the assignment.
The data is going to tell you the assignment worked, when what worked was parents breaking the assignment down into component parts and teaching each part separately.
..................................
Beyond this, why am I reading about summer camp?
Miller quotes an NCES study, for instance, which found that "42.5 percent of children in high-income households attended camp the summer after kindergarten, compared with just 5.4 percent of children in low-income" families.
Are these researchers suggesting that middle class kids routinely gain 47 points on reading tests at camp?
(How much is 47 points, anyway? How many points did kids gain during the school year? I may have to see if Ed can get a copy of the article...)
If summer camps run by distracted teenagers are producing major gains in reading, maybe we need some studies of the super-effective teaching methods known only to 18 year old, untrained college kids.
..................................
Speaking of camp, C. and I played hooky Wednesday (though, as C. pointed out, you can't really play hooky from camp) and went to the city to see Summercamp!
You must see it.
Summercamp! is a laugh-cry-embrace-life sort of movie; I don't think I've cried so much in a movie theater since seeing Forrest Gump two weeks after giving birth to twins. [for newbies: my oldest son Jimmy, who was then age 7, is autistic]
An incredible movie - beautiful. There's no crying 'til the very end, and even then it's good crying. I promise.
from the NY Sun review:
In the summer of 2003, filmmakers Bradley Beesley and Sarah Price joined forces to tackle a documentary subject of almost unbearably powerful emotions and compulsively watchable conflict: a season at a Midwestern sleepover camp.
[snip]
The film's two stars are Holly, a charismatically energetic and wistful girl, and Cameron, an overweight kid with an unusual flair for challenging counselors' patience and making enemies among his peers.
[snip]
Holly and Cameron "are our main characters because they related more to adults and adult-type issues and they didn't have a whole lot of friends within their cabins," Mr. Beesley said.
[snip]
Something else the filmmakers discovered was how much prescription medication has permeated children's lives. "There was this group of kids going to the nurse's office every night," Ms. Price said. "It took us a few days to catch on to what was going on." Per their parent's wishes, campers diagnosed with attention deficit disorder and other behavioral maladies were reporting to receive their prescribed meds. Images in "Summercamp!" of what appear to be perfectly healthy children lining up to swallow pills like the mental patients in "One Flew Over the Cuckcoo's Nest" are disturbing; at the same time, a scene in which one boy flirts with a girl by bragging about the magnitude of his ADD is hilarious.
[snip]
"Summercamp!" also doggedly follows Cameron down a road of trials littered with obstacles of the boy's own devising. Cameron has an unfortunate genius for clumsily rebellious behavior; witnessing his steady failures and occasional triumphs will likely empower the inner outcast in anyone. "We're watching two kids go through growing pains and be open enough about it to sort of let us discover and experience it while they do," Ms. Price said.
Pure pleasure.
My dean teaches math to fifth graders
Thursday, July 19, 2007
rightwingprof on what students don't know
I'm supposed to be racking up more Time On Task on my super-duper self-regulation anti-procrastination chart, but first this message from the future:
Ed discovered yesterday that C. doesn't have a clue what a 10% reduction in price means.
He couldn't think how to figure it, and, when Ed reminded him how to figure it (he does know the procedures), he didn't know what the answer meant.
Ed reminded him about moving the decimal point. C. moved it the wrong way and came up with the possibility that the new cost would be $350. (How many times have I told him - and had him tell me - that when you "move the decimal point" by one digit you are either multiplying or dividing by ten, depending upon which way you moved it? Many.)
When he eventually figured out that 10% was $3.50, he got confused because he thought $3.50 must be the reduced price and he knew that couldn't be right. (Thank God for small favors.)
He had no idea he needed to subtract the 10% from the original price, though he did eventually realize there was a second step. (Next question: how many times have I told him - and had him tell me - that to find out what the price will be after a 10% deduction you can either multiply the original price by 0.1 and subtract the product from the original price, OR you can multiply the original price by 0.9 and be done with it? Many.)
This reminds me of my friend's son who, in 8th grade this year, could not figure a 10% tip for a pizza delivery - not even when his mom gave him pencil and paper and told him to do it that way.
He's in the accelerated math class, too.
These kids have learned nothing.
It's a nightmare.
Speaking of which, Susan J asked the other day whether it might make more sense to start with fractions this summer, instead of percent.
The answer is yes.
I've put away Algebra 1, and I've fished out my copy of Saxon 7/6 (7th grade), which it turns out I do own, after all.
We're going to be doing Saxon bar models for the rest of the summer and then on into the school year and possibly beyond......right up to the point at which C. has fractions, decimals, and percents imprinted on his tough, leathery, little pre-teen brain.
Maybe a branding iron would do the trick.
.........................
That wasn't a very nice thing to say.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux: Robert Slavin on transfer of knowledge
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
birthday and a vacation
But forget "higher-order thinking." Let's turn to basic mathematical knowledge that every sixth-grader should know, but many of my students (more and more each semester) do not. And I know they don't know these things because I have to explain them in class. Students do not know
- what a rate is: I have more than a few students who do not understand why they cannot just add the tax rate to the item price to get the total sale price.
- basic addition and subtraction: I have more than a few students who do not understand that you subtract the cost from the revenue to get the gross profit margin, or do not know that to get the total costs, you add the fixed and variable costs.
- basic multiplication and division: I have more than a few students who do not know that they must mutiply the number of units by the unit cost to get the total cost. I have more than a few students who do not know that because the interest rate is annual, they must divide it by 12 to calculate the monthly amoritization table.
- the relationship between multiplication and division: When we start doing optimization problems in Excel Solver, I have to tell students that because Solver does not like division, they must construct their problem with multiplication instead, and I have many students who do not know or understand how to do this (I also have more than a few students who do not know that you cannot divide by zero.)
- what an arithmetic mean is: I have more than a few students who not only do not understand what a mean is, but seem unable to grasp the concept. It goes without saying that they also do not grasp any statistical concept beyond the arithmetic mean.
Ed discovered yesterday that C. doesn't have a clue what a 10% reduction in price means.
He couldn't think how to figure it, and, when Ed reminded him how to figure it (he does know the procedures), he didn't know what the answer meant.
Ed reminded him about moving the decimal point. C. moved it the wrong way and came up with the possibility that the new cost would be $350. (How many times have I told him - and had him tell me - that when you "move the decimal point" by one digit you are either multiplying or dividing by ten, depending upon which way you moved it? Many.)
When he eventually figured out that 10% was $3.50, he got confused because he thought $3.50 must be the reduced price and he knew that couldn't be right. (Thank God for small favors.)
He had no idea he needed to subtract the 10% from the original price, though he did eventually realize there was a second step. (Next question: how many times have I told him - and had him tell me - that to find out what the price will be after a 10% deduction you can either multiply the original price by 0.1 and subtract the product from the original price, OR you can multiply the original price by 0.9 and be done with it? Many.)
This reminds me of my friend's son who, in 8th grade this year, could not figure a 10% tip for a pizza delivery - not even when his mom gave him pencil and paper and told him to do it that way.
He's in the accelerated math class, too.
These kids have learned nothing.
It's a nightmare.
Speaking of which, Susan J asked the other day whether it might make more sense to start with fractions this summer, instead of percent.
The answer is yes.
I've put away Algebra 1, and I've fished out my copy of Saxon 7/6 (7th grade), which it turns out I do own, after all.
We're going to be doing Saxon bar models for the rest of the summer and then on into the school year and possibly beyond......right up to the point at which C. has fractions, decimals, and percents imprinted on his tough, leathery, little pre-teen brain.
Maybe a branding iron would do the trick.
.........................
That wasn't a very nice thing to say.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux: Robert Slavin on transfer of knowledge
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
birthday and a vacation
housekeeping
I've scanned & posted the percent ovals Saxon Algebra 1 (& Algebra 1/2, I believe) uses for problems in which the quantity increases.
Back later ---
Back later ---
New Where's the Math Video
Where's the Math has a new video, which is about 20 minutes but worth watching. It focuses on the schools in Bellevue, Washington. Schools in Bellevue score high on Jay Mathews' index, but this video tells a side of the story that isn't publicized very well--and it should be.
Go here and the video is labeled "Math Education in Bellevue, WA"
Go here and the video is labeled "Math Education in Bellevue, WA"
Wednesday, July 18, 2007
Singapore & TIMSS
Regarding the Timms studies, Barry mentioned:
"the accusation is that only the top students are tested. That leaves open the question about 4th grade.But I would appreciate any light you could shed on that."
Here's some info on validity from the TIMSS website:
Just a note that I heard echoed from several of the summer math program attendees. The Japanese mathematics curriculum is just as strong as Singapore's. Singapore syllabus wins because it's already in English.How can we be sure the data is comparable?
The International Study Center at Boston College works to ensure that data collection procedures across countries are comparable. To this end, the International Study Center institutes the following procedures for quality assurance:
- Coordinated by the TIMSS Sampling Referee, national school and student samples are rigorously reviewed for bias and international comparability.
- Utilizing two independent translations within each country, the TIMSS materials are translated into the national languages of the participating countries. Once these translations are reconciled, the International Study Center verifies these results through the use of a professional translation agency.
- National Research Coordinators (NRCs) and their staff are thoroughly trained in data collection and scoring procedures at international conferences designed specifically for this purpose. The TIMSS International Study Center then continues to monitor the work of the NRCs and their staff for scoring reliability.
- Site visits by quality control staff are conducted during the testing period to further ensure the international data collection procedures are being followed at the national level.
- Finally, an extensive review of data is conducted for internal and cross-country consistency.
Tuesday, July 17, 2007
Singapore Math Observations pt. 2
Before heading to Singapore, I solicited questions from the KTM blog and teaching friends. Here are some answers! Feel free to check out some photos of Singapore schools and their unusual fruit at my flickr site.
Numbers on this varied, depending on who you asked. I chatted with a group of secondary students and sec. 3 kids (That would be our grade 9) received very little, while there was a much higher amount getting tutoring in sec. 4 (grade 10). Sec. 4 students take their “0” levels in October and their score dictates their future education. Sec. 4 students were serious in class and talked of having little life outside of school. Primary student percentages of students getting tutored ranged from 35 out of 40, to minimal. These answers came from a music teacher, maths teachers, principals and professors at the National Institute of Education. Parents pay for this tutoring. Expectations in Singapore are very high.
What do Singapore teachers say about how to teach place value?
Sorry, never got to this.
Is math a special in their elementary schools? In other words, do elementary school teachers of math have to be licensed specifically in math?
Elementary or Primary teachers are certified in maths, English and a third subject, usually science. Perhaps this is a good place to note that science doesn’t get tested and thusly, taught as a subject until P4 (4th grade). Core subjects in P1-P3 are maths, English and Mother Tongue.
Get all the details you can about their once a week meetings, in which they discuss math. That's so in contrast with American teachers who meet to talk about child development.
Wait until you hear about this: Singapore teachers get 100 hours a year of “upgrading” (continuing ed.) They may take calligraphy classes, further their own education, participate in teacher discussion sessions or lesson study. Since they only teach 3 core subjects a day, they have the time AND they have offices. Every school we visited had a room with teacher cubicles so they had their own place to grade papers, plan, etc., outside of the classroom. Of course, they are expected to tutor after school or participate in CCA.
The gap in learning speed is largest as kids begin learning a subject, which I believe means that the gap should be largest in 1st grade. However, they're keeping kids together in 1st through 4th grades.
So how does this happen?
This is truly remarkable. They begin pullout remediation in the 1st grade to ensure that students can read and do maths. This may happen during the classroom day during PE or another subject or it may happen in Co-Curricular Activities time. See my previous post for more on the special needs areas.
Are the parents simply able to bring the slower kids along through extra practice & reteaching at home? Do the schools advise parents of slower learners to provide extra practice?
Of course they advise parents that their kids are not performing up to snuff. Parental assistance is both a blessing and a burden (as any teacher who has seen algebra on a 3rd grader’s paper knows). Since the students are at school as late as 6pm some nights, there appears to be plenty of time for basic practice in maths. Another aside on parents helping in Singapore: The Primary Mathematics syllabus came out of th Ministry of Education in 1996. Parents were not taught using this curriculum, if they were taught mathematics beyond simple computation at all.
Do they advise parents to sign their kids up for KUMON? RE: KUMON - what is their attitude towards KUMON? Do schools ever coordinate with KUMON (or other tutoring businesses)?
They may advise extra tuition (as tutoring is called). Kumon is trying to break into the country, but is fairly unsuccessful. Schools do not coordinate with outside tutors.
I'm interested in what they see students' "sticking points" as. (fractions? long division? absolute value & operations with integers?)
Good question and they could certainly tell you. We forgot to ask.
Do they teach calculus in high school? I gather they don't - if not, why not?
They teach calculus in Junior College, which is the equivalent of our 11th & 12th grades.
How much time is spent per day/year/week whatever on math for a typical Singapore schoolkid (answer may be different per grade, ya?)? I'd include in-school, Kumon, at home, etc.
5.5 to 6 hours per week in primary grades, depending on the school. remediation and after school are hard to gauge. Many parents pile homework onto their children without any suggestion from the schools. Again, primary teachers we talked to in Singapore view homework as most teachers in the US and assign very little. Finding algebra on a 3rd graders homework in adult handwriting is not just a U.S. problem. (Although, we don't usually have maids that do it, like they do in Singapore!)
How much drill do they do? I've been supplementing the textbooks and workbooks with drill. I understand that this is normal. But ... how much is expected/typical?
No set numbers on this, but they are moving away from drill & kill and towards more hands-on lessons. Children actually beg for maths workbooks and there are hundreds to choose from at the local stationary store, Popular. I have no shame in telling you that we had to buy an extra suitcase to keep our luggage under 22kg per bag due to all the books we purchased.
How do they integrate technology?
Very, very well, from what I saw. Every school gets “upgrading” every 6 years. Cedar Primary is the IT showcase for the country, but Guanyang Primary was just as impressive, they had a green screen room and keyboards attached to desktops for composing! Teachers have all been trained in IT and used it seamlessly in their lessons. Most schools have a bank of powerpoint maths lessons available.
All students in the country have access to hi-speed internet. At the one secondary school, they stated home use rates of 95%, with 5% of those homes being subsidized. The other 5% don’t believe in the internet or don’t want their child addicted to video gaming, which is a major “behavior” problem (As one Principal termed it)
A truly minor point, has Singapore looked at/purchased/implemented any of the reform math programs circulating through the US school systems?
No. American textbooks are not approved by the Ministry of Education and cannot be bought with government funds.
The director of curriculum told me quite smugly that "They use Everyday Math in Singapore"
The Singapore American School has recently selected Everyday Math for its curriculum. They are a separate entity, designed to teach Americans living in Singapore and can teach as they choose, since they aren't receiving government funding.
Questions & Answers
Numbers on this varied, depending on who you asked. I chatted with a group of secondary students and sec. 3 kids (That would be our grade 9) received very little, while there was a much higher amount getting tutoring in sec. 4 (grade 10). Sec. 4 students take their “0” levels in October and their score dictates their future education. Sec. 4 students were serious in class and talked of having little life outside of school.
What do Singapore teachers say about how to teach place value?
Sorry, never got to this.
Is math a special in their elementary schools? In other words, do elementary school teachers of math have to be licensed specifically in math?
Elementary or Primary teachers are certified in maths, English and a third subject, usually science. Perhaps this is a good place to note that science doesn’t get tested and thusly, taught as a subject until P4 (4th grade). Core subjects in P1-P3 are maths, English and Mother Tongue.
Get all the details you can about their once a week meetings, in which they discuss math. That's so in contrast with American teachers who meet to talk about child development.
Wait until you hear about this: Singapore teachers get 100 hours a year of “upgrading” (continuing ed.) They may take calligraphy classes, further their own education, participate in teacher discussion sessions or lesson study. Since they only teach 3 core subjects a day, they have the time AND they have offices. Every school we visited had a room with teacher cubicles so they had their own place to grade papers, plan, etc., outside of the classroom. Of course, they are expected to tutor after school or participate in CCA.
The gap in learning speed is largest as kids begin learning a subject, which I believe means that the gap should be largest in 1st grade. However, they're keeping kids together in 1st through 4th grades.
So how does this happen?
This is truly remarkable. They begin pullout remediation in the 1st grade to ensure that students can read and do maths. This may happen during the classroom day during PE or another subject or it may happen in Co-Curricular Activities time. See my previous post for more on the special needs areas.
Are the parents simply able to bring the slower kids along through extra practice & reteaching at home? Do the schools advise parents of slower learners to provide extra practice?
Of course they advise parents that their kids are not performing up to snuff. Parental assistance is both a blessing and a burden (as any teacher who has seen algebra on a 3rd grader’s paper knows). Since the students are at school as late as 6pm some nights, there appears to be plenty of time for basic practice in maths. Another aside on parents helping in Singapore: The Primary Mathematics syllabus came out of th Ministry of Education in 1996. Parents were not taught using this curriculum, if they were taught mathematics beyond simple computation at all.
Do they advise parents to sign their kids up for KUMON? RE: KUMON - what is their attitude towards KUMON? Do schools ever coordinate with KUMON (or other tutoring businesses)?
They may advise extra tuition (as tutoring is called). Kumon is trying to break into the country, but is fairly unsuccessful. Schools do not coordinate with outside tutors.
I'm interested in what they see students' "sticking points" as. (fractions? long division? absolute value & operations with integers?)
Good question and they could certainly tell you. We forgot to ask.
Do they teach calculus in high school? I gather they don't - if not, why not?
They teach calculus in Junior College, which is the equivalent of our 11th & 12th grades.
How much time is spent per day/year/week whatever on math for a typical Singapore schoolkid (answer may be different per grade, ya?)? I'd include in-school, Kumon, at home, etc.
5.5 to 6 hours per week in primary grades, depending on the school. remediation and after school are hard to gauge. Many parents pile homework onto their children without any suggestion from the schools. Again, primary teachers we talked to in Singapore view homework as most teachers in the US and assign very little. Finding algebra on a 3rd graders homework in adult handwriting is not just a U.S. problem. (Although, we don't usually have maids that do it, like they do in Singapore!)
How much drill do they do? I've been supplementing the textbooks and workbooks with drill. I understand that this is normal. But ... how much is expected/typical?
No set numbers on this, but they are moving away from drill & kill and towards more hands-on lessons. Children actually beg for maths workbooks and there are hundreds to choose from at the local stationary store, Popular. I have no shame in telling you that we had to buy an extra suitcase to keep our luggage under 22kg per bag due to all the books we purchased.
How do they integrate technology?
Very, very well, from what I saw. Every school gets “upgrading” every 6 years. Cedar Primary is the IT showcase for the country, but Guanyang Primary was just as impressive, they had a green screen room and keyboards attached to desktops for composing! Teachers have all been trained in IT and used it seamlessly in their lessons. Most schools have a bank of powerpoint maths lessons available.
All students in the country have access to hi-speed internet. At the one secondary school, they stated home use rates of 95%, with 5% of those homes being subsidized. The other 5% don’t believe in the internet or don’t want their child addicted to video gaming, which is a major “behavior” problem (As one Principal termed it)
A truly minor point, has Singapore looked at/purchased/implemented any of the reform math programs circulating through the US school systems?
No. American textbooks are not approved by the Ministry of Education and cannot be bought with government funds.
The director of curriculum told me quite smugly that "They use Everyday Math in Singapore"
The Singapore American School has recently selected Everyday Math for its curriculum. They are a separate entity, designed to teach Americans living in Singapore and can teach as they choose, since they aren't receiving government funding.
Singapore Math Observations pt. 1
Here are answers to some questions posted on the KTM blog about Singapore Maths. Opinions on this paper belong only to program attendee Tricia Salerno, a Singapore Maths Coach and the author, Cassandra Turner.
A brief overview of the Singapore educational system:
The system sounds more complicated than it probably is. Visit this page at the Ministry of Education for a nice graphic that shows student options at every level. We noticed several important differences between the US and Singaporean education systems. Perhaps the single most important difference is that the government and people of Singapore view education as an investment in their future. The country’s only natural resource is their citizens and they spend the money to create great citizens. In America, education is viewed as an expense.
Primary
There is no public kindergarten in Singapore. It may be provided as day care, through churches or the political parties. Because of this, students enter P1 with a wide variation of prior knowledge. Remediation begins in P1. Primary students begin their school days either at 7:30 or 8 am and are released around 1:00pm. They buy or return home for lunch, then come back to school for Co-Curricular Activities (CCA). These may include band, sports, tuition (tutoring), dance robotics, video or anything we might consider an “after school activity” See http://www.moe.gov.sg/ccab/ for more information. My conference partner and I got the impression that a certain number of hours in CCAs were required weekly, but it’s possible that this is a school requirement, not a national standard. Remediation is strongly encouraged for certain students and we got the sense that a teacher’s recommendation of tuition (tutoring or remediation) is always heeded.
Because all students are ELL, the emphasis in the first 3 years of primary school is on English, maths and Mother Tongue (Tamil, Mandarin or Malay). In maths, students are ability grouped (called “subject banding”) into 3 groups. Principals refer to it as HAMALA – High Ability, Middle Ability, and Low Ability. Science and social sciences are integrated, but not considered “core” subjects. Science becomes a core subject beginning in P4 (4th grade), however, we found many examples of strong integration in the classrooms we visited (two P1 classes, one P3, two P4). Students sit for the Primary School Leaving Exam (PSLE) at the end of 6th grade. This determines their placement in Secondary School. Student capacity is capped at 40 students in a regular classroom from P3 and up, 30 students for P1 & P2. A music teacher told me she had 80 in her classes.
Info on the change from streaming to subject banding from the MOE website:
SUBJECT-BASED BANDING IN PRIMARY SCHOOL
Key changes
| > | Starting from the 2008 Primary 5 cohort, primary schools will introduce Subject-based Banding to replace the current EM3 stream. Currently EM3 stream students offer the Foundation level for all subjects. With Subject-based Banding, students will be able to offer a mix of Standard or Foundation subjects depending on their aptitude in each subject. For instance, if a student is weak in English and Mathematics, he can choose to take English and Mathematics at the Foundation level while taking Mother Tongue Language and Science at the Standard level. |
Students in the top 1% of ability, based on testing are offered the chance to study at a special school for the gifted.
Below is a diagram of Special Education system for Primary students (Click it to get a better image):
Secondary
Compulsory education is 6 years, so it is possible that some students do not go on with their education beyond P6. Students must submit to several interviews with parents and the principal before they are allowed to end their schooling at this point. Secondary students are placed in a track based on their PSLE. There is the Express Course, Normal Course (Academic) and the Normal Course (Technical) and from there it gets confusing. Try the link under “brief overview” above to the MOE graphic.
During our trip, we visited two secondary schools. Between the two, we observed two Sec. 1 maths classes (grade 7) and a Sec.4 (grade 10) maths class. What we found is that some things are similar the world over. We saw bored students, disruptive students and teachers giving it their all. We also saw highly engaged students, who had high expectations of themselves and their school. Although Singapore no longer ranks schools by their achievement, both schools we visited were still considered mid-tier schools.
The principal at Kuo Chuan Presbyterian Secondary School told us that he still canes students for disruptive behavior in the classroom. It is still allowed in Singapore, but only the Principal may do so and he would never do it publicly, just in front of the class the student had disrupted. We didn’t hear about anyone else still caning, but were surprised to hear about it from the principal at the last school we visited. (Put that down as a topic we wished we had thought to ask about sooner.)
Students take the “O” level exams towards the end of Sec. 4. Normal (academic) students have a 5th year before they take the test. Their score determines if they go on to Junior College (Grades 11 & 12) or a technical school. Many Secondary schools are now affiliated with Junior Colleges. Students in the Express Course can bypass the tests and go straight on to the JC. Maths students that we saw were additionally sorted by “E” maths and “A” maths. The “A” maths consists of an additional course on top of the “E” maths course and is recommended for students going on to any type of engineering or technically advanced coursework. Again, refer to the picture on the MOE website if your head is now spinning.
A bit about the teaching profession:
Teachers in Singapore are valued right below doctors & lawyers, although not paid nearly as well. A teacher just starting out begins at around $24,000 a year after mandatory savings of 7% is taken out. To become a teacher, you must first get a job from the Ministry of Education, and then go to school to get your degree or diploma. After that you may teach full-time, although you are working part time in a classroom while in school. Teachers who have difficulty are immediately given remediation as a poor teacher reflects a poor principal and no principal wants their teacher to be a failure. Teachers only teach three core subjects in the lower primary and four in P4-6. That allows them time to grade every piece of paper that a student turns in, and thoroughly at that. Even with 40 kids in a classroom, I can tell that they have a better grasp on each student as an individual than most teachers in the US.
back to basics
hmmm...
After reading this passage in Carl Binder's article Doesn't Everybody Need Fluency? (pdf file) I decided to give C. a speed test today.
70 problems per minute = 70 problems per 60 seconds
C. did two timed tests. The first was 50 problems; the second was 25.
70 problems/60 seconds = 50 problems/43 seconds
To meet this performance, standard he needed to write answers to 50 simple addition problems in 43 seconds. Right?
First test: 50 problems in 190 seconds.
yikes
After he was done, I took the same test & clocked in at 50 problems in 35 seconds without breaking a sweat. (Try it yourself. You'll see.)
I had C. try the test again. He cut his time in half, but he was still at 50 problems/66 seconds. He says he's tired & has a headache....but then again I'm tired, too, (albeit sans headache) not to mention old.
I'll have him take a third speed test tomorrow. If he's not down to 43 problems/50 seconds, I'll test his speed writing digits. Then, when I find out there's no way in h - e - double hockey sticks he can write 100 digits in 60 seconds, we will commence printing practice.
So.... we're back to handwriting. Yet another inconsequential non-21st century skill never, ever taught to mastery in our public schools!
bonus narrative: C. used cursive writing to label his Saxon percent ovals, leading me to the discovery that he's writing his f's wrong.
"I think you're writing your f's wrong."
"No I'm not! That's how you're supposed to write 'f'!"
I decided not to argue about it.
Let off the hook, C. stared at my cursive version of the letter "b" for a couple of seconds, then said, "I forgot how to write b's."
I decided not to argue about that, either.
simple addition worksheets online:
create & print whole number addition worksheets from aplusmath (best source - you can specify 50 problems)
free whole number addition worksheets from S&S Software
create & print addition worksheets
free math worksheets from tlsbooks
free math worksheets from math-drills.com
back to basics
speed test
After reading this passage in Carl Binder's article Doesn't Everybody Need Fluency? (pdf file) I decided to give C. a speed test today.
[I]n regular classrooms we learned that students need to be able to write answers to between 70 and 90 simple addition problems per minute in order to be able to successfully and smoothly master arithmetic story problems. However, some students seemed to level off at around 20 or 30 problems per minute, and no amount of reward or encouragement seemed to help. Some of our colleagues (Starlin, 1971; Haughton, 1972) decided to check how many digits those students could read and write per minute—critical components of writing answers to problems. As you might guess, they were very slow, which held down their composite performance. With practice of the components on their own to the point of rapid accurate performance (for example, reading and writing digits at 100 per minute or more), students were able to progress smoothly toward competence on solving the written math problems.
70 problems per minute = 70 problems per 60 seconds
C. did two timed tests. The first was 50 problems; the second was 25.
70 problems/60 seconds = 50 problems/43 seconds
To meet this performance, standard he needed to write answers to 50 simple addition problems in 43 seconds. Right?
First test: 50 problems in 190 seconds.
yikes
After he was done, I took the same test & clocked in at 50 problems in 35 seconds without breaking a sweat. (Try it yourself. You'll see.)
I had C. try the test again. He cut his time in half, but he was still at 50 problems/66 seconds. He says he's tired & has a headache....but then again I'm tired, too, (albeit sans headache) not to mention old.
I'll have him take a third speed test tomorrow. If he's not down to 43 problems/50 seconds, I'll test his speed writing digits. Then, when I find out there's no way in h - e - double hockey sticks he can write 100 digits in 60 seconds, we will commence printing practice.
So.... we're back to handwriting. Yet another inconsequential non-21st century skill never, ever taught to mastery in our public schools!
bonus narrative: C. used cursive writing to label his Saxon percent ovals, leading me to the discovery that he's writing his f's wrong.
"I think you're writing your f's wrong."
"No I'm not! That's how you're supposed to write 'f'!"
I decided not to argue about it.
Let off the hook, C. stared at my cursive version of the letter "b" for a couple of seconds, then said, "I forgot how to write b's."
I decided not to argue about that, either.
simple addition worksheets online:
create & print whole number addition worksheets from aplusmath (best source - you can specify 50 problems)
free whole number addition worksheets from S&S Software
create & print addition worksheets
free math worksheets from tlsbooks
free math worksheets from math-drills.com
back to basics
speed test
young lives destroyed by failure to learn dimensional analysis
"I was a Wilton High School student who dozed off while Mr. Laptick taught us dimensional analysis in physical science. I never quite got the hang of it. It irritated me... all of those fractions. I never really liked fractions. Although my grades had been pretty high, I got a D in physical science and subsequently dropped out of chemistry in the first quarter of my junior year. It was not long before I started on drugs, and then crime to support my drug habit. I have recently learned dimensional analysis and realize how simply it could have solved all of my problems. Alas, it is too late. I won't get out of prison until 2008 and even then, my self image is permanently damaged. I attribute all of my problems to my unwillingness to learn dimensional analysis." Jane
"I thought I knew everything and that sports was the only thing that mattered in high school. When Mr. Hoogenboom taught our class dimensional analysis, I didn't care about it at all. I was making plans for the weekend with my girlfriend who loved me because I was a running back and not because of physical science. While other kids were home solving dimensional analysis problems, I was practicing making end sweeps. Then one day I was hit hard. Splat. My knee was gone. I was despondent. My girl friend deserted me. My parents, who used to brag about my football stats, started getting on my case about grades. I decided to throw myself into my school work. But I couldn't understand anything. I would get wrong answers all of the time. I now realize that my failure in school came from never having learned dimensional analysis. Alas, I thought everyone else was smarter. After the constant humiliation of failing I finally gave up. I am worthless. I have no friends, no skills, no interests. I have now learned dimensional analysis, but it is too late." Bill
I was at home, sick with the flu when Mr. Mycyk taught my class about Dimensional Analysis. Despite opportunities given to me to make up the assignments that I had missed, I chose to not do them. I thought that my mathematical abilities were already sufficient. How wrong I was! It’s been five years since I took that class--Now I spend my afternoons panhandling at traffic lights, hoping for passersby to give me spare change. If I ‘m lucky enough to scam a buck after a day’s work, I’m still not sure if my hourly rate makes cents. --Mario
source:
Dimensional Analysis
dimensional analysis problems from ktm-1
You may have to hit refresh a couple of times.
Dan K on dimensional anlysis
dimensional dominoes from Dan K
dimensional analysis worksheets from Dan K
a way to teach unit conversion (Carolyn Johnston)
teaching Christopher unit multipliers
dimensional analysis word problems and answers
another cool dimensional analysis problem
solution
dimensional analysis problem from Math Forum
Dr. Ian talks about fractions and units
another triumph for dimensional analysis
dimensional analysis emergency
Dan K on dimensional anlysis
dimensional dominoes from Dan K
dimensional analysis worksheets from Dan K
a way to teach unit conversion (Carolyn Johnston)
teaching Christopher unit multipliers
dimensional analysis word problems and answers
another cool dimensional analysis problem
solution
dimensional analysis problem from Math Forum
Dr. Ian talks about fractions and units
another triumph for dimensional analysis
dimensional analysis emergency
teach your children unit multipliers....
from Gary Carson:
I guffawed (inwardly - I do not guffaw out loud) when I read Gary's comment.
This is exactly the way I felt when I first laid eyes on unit multipliers and figured out what they were. (For the uninitiated, the single best place on the web to look at unit multipliers is Donna Young's homeschool website. Click on "Math" at the left. Then click on "Unit Multipliers" at the bottom right.)
Ed's cousin, a chemistry teacher at a high performing high school in IL (has a Ph.D. in chemstry) told us that a lot of his students come into his class not having the first clue about fractions or ratios. The best students do, he said, but no one else.
So the question is: is he going to teach remedial math, or is he going to teach chemistry?
He teaches them chemistry and unit multipliers.
I managed to pass both intro chemistry and intro physics in college without learning anything at all about either subject just by being able to manipulate dimensions to arrive at an answer for exam questions. In one semester of Chem I even made a B and had no clue about the actual subject matter.
I guffawed (inwardly - I do not guffaw out loud) when I read Gary's comment.
This is exactly the way I felt when I first laid eyes on unit multipliers and figured out what they were. (For the uninitiated, the single best place on the web to look at unit multipliers is Donna Young's homeschool website. Click on "Math" at the left. Then click on "Unit Multipliers" at the bottom right.)
Ed's cousin, a chemistry teacher at a high performing high school in IL (has a Ph.D. in chemstry) told us that a lot of his students come into his class not having the first clue about fractions or ratios. The best students do, he said, but no one else.
So the question is: is he going to teach remedial math, or is he going to teach chemistry?
He teaches them chemistry and unit multipliers.
dimensional analysis advice from Susan J
As a former chemistry teacher I would say you cannot overemphasize an understanding of dimensional analysis.
For kids who don't see the point, ask them "backwards" questions such as how many feet are in an inch?
Also, have them do long chains such as determining how many centimeters in a mile. It's good to have figured these out in advance yourself so your student is instantly rewarded if they get the right answer.
Saxon Algebra 1: how to teach percent word problems
from Saxon Algebra 1, p 236:
large image here
.............................
The first time C. tried this problem he found it quite difficult. (And, yes, we're talking about a kid who is 1/3 of the way through Math A: algebra 1/geometry.)
Fortunately I stumbled upon the precision teaching folks at that point, and realized I needed to teach the component skills separately. In this case, C. needed practice drawing and labeling the diagram, so I had him do only that for 3 sessions, I think.
Then we did word problems like the one above.
We moved to "story" problems accidentally, "story" problems meaning:
"Jane and Faye have 32 bagatelles left. If they began with 160 bagatelles, what percent of the original number remains?"
(I managed to assign a story problem accidentally because I was flipping through the solution manual looking for solutions with ovals in them, and didn't realize the solution I'd found was a solution to a story problem, not a word problem. fyi)
C. didn't recognize that the story problem could be solved using the same ovals and percent equations he uses to solve percent word problems. Talk about hyperspecificity. I was so wrong to say autistic people and animals are hyperspecific and the rest of us aren't. God is punishing me.*
Today I need to locate the first Saxon lesson on percent story problems and teach that directly.
Nevertheless, in spite of my bumbling, this method is slowly but surely leading C. to some comprehension of what is happening when you take a percent of something in the real world, if you'll pardon the expression.
Speaking of the real world, what is a bagatelle?
I'll post a screenshot of the oval diagrams he uses for problems in which the solution is more than 100% later.
.............................
update: Saxon oval diagrams for problems in which the solution is greater than 100% of the original quantity
large image here
* I don't say that with disrespect. God should punish me.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux: Robert Slavin on transfer of knowledge
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
To solve word problems about percent, it is necessary to be able to visualize the problem. We will begin to work on achieving this visualization by drawing diagrams of percent problems after we work the problems. Learning to draw these diagrams is very important.
Twenty percent of what number is 15? Work the problem and then draw a diagram of the problem.
We will use ... 20 for percent, WN for what number, and 15 for is.
20/100 · WN = 15
The "before" diagram is 75, which represents 100 percent. The "after" diagram shows that 15 is 20 percent. Thus the other part must be 60, which is 80 percent.
large image here
.............................
The first time C. tried this problem he found it quite difficult. (And, yes, we're talking about a kid who is 1/3 of the way through Math A: algebra 1/geometry.)
Fortunately I stumbled upon the precision teaching folks at that point, and realized I needed to teach the component skills separately. In this case, C. needed practice drawing and labeling the diagram, so I had him do only that for 3 sessions, I think.
Then we did word problems like the one above.
We moved to "story" problems accidentally, "story" problems meaning:
"Jane and Faye have 32 bagatelles left. If they began with 160 bagatelles, what percent of the original number remains?"
(I managed to assign a story problem accidentally because I was flipping through the solution manual looking for solutions with ovals in them, and didn't realize the solution I'd found was a solution to a story problem, not a word problem. fyi)
C. didn't recognize that the story problem could be solved using the same ovals and percent equations he uses to solve percent word problems. Talk about hyperspecificity. I was so wrong to say autistic people and animals are hyperspecific and the rest of us aren't. God is punishing me.*
Today I need to locate the first Saxon lesson on percent story problems and teach that directly.
Nevertheless, in spite of my bumbling, this method is slowly but surely leading C. to some comprehension of what is happening when you take a percent of something in the real world, if you'll pardon the expression.
Speaking of the real world, what is a bagatelle?
I'll post a screenshot of the oval diagrams he uses for problems in which the solution is more than 100% later.
.............................
update: Saxon oval diagrams for problems in which the solution is greater than 100% of the original quantity
When a problem discusses a quantity that increases, the final quantity is greater than the initial quantity. If we let the initial quantity represent 100 percent, the final percent will be greater than 100. This means that the "after" diagram representing the final quantity will be larger than the "before" diagram. The "after" diagrams in this book will not be drawn to scale. [emphasis in the original]
[snip]
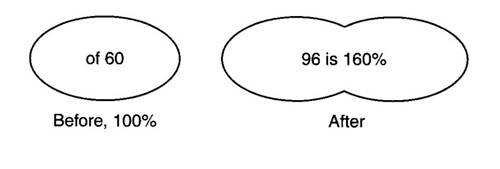
What number is 160 percent of 60? Work the problem and then draw a diagram of the problem.
WN = 160/100 · 60
large image here
* I don't say that with disrespect. God should punish me.
hyperspecificity in autism
hyperspecificity in autism and animals
hyperspecificty in the rest of my life
hyperspecificity redux: Robert Slavin on transfer of knowledge
Inflexible Knowledge: The First Step to Expertise
Devlin on Lave
rightwingprof on what college students don't know
percent troubles
what is 10 percent?
Vicky S on Warriner's
My old Warriner's (Revised Edition with Supplement, Complete Course, Copyright 1969) is still my single source for writing and grammar. You could plan your whole course of home study from just the contents, although nothing beats having the book. The amazing thing about Warriner's is that in addition to having all the following information, it has hundreds of examples and exercises, all packed into this paperback size hard cover of 800+ pages. Here are the contents followed by some brief excerpts from the preface.
Contents:
Part One: Grammar
Parts of Speech, Parts of a Sentence, Phrase, Clause
Part Two: Usage
Levels of Usage (Standard vs. Substandard English), Agreement, Pronouns, Verbs, Modifiers
Part Three: Composition: Sentence Structure
Sentence Completeness, Coordination/Subordination, Clear Reference, Placement of Modifiers, Parallel Structure (really important!), Unnecessary Shifts in Sentences, Sentence Conciseness, Sentence Variety, Effective Diction, Exercises in Sentence Revision
Part Four: Composition: Paragraphs and Longer Papers
The Effective Paragraph, Expository Writing, Language and Logic, Exercises in Composition, Research Paper, Letter Writing
Part Five: Aids to Good English
Information in the Library, Reference Books, Dictionary, Vocabulary
Part Six: Speaking and Listening
Discussion and Debate, Effective Speech
Part Seven: Mechanics
Capitalization, Punctuation, Manuscript Form, Spelling
Part Eight: A New Look at Grammar
Structural and Transformational Grammars
College Entrance and Other Examinations
Tests of Verbal Aptitude, Composition Tests
Supplement
Making Writing Interesting
From the Preface (citation above):
"The teacher of senior English occupies a difficult but challenging position. Because the course he teaches is in large part a summary of all the English courses that have preceded it, he feels obligated to review, or reteach, everything. Two thoughts impress upon him the magnitude of his responsibilities. The first is the image of the college English instructor lurking in the future of his college-bound students, ready and, it often seems, eager to find weaknesses in their high school preparation. The other is the even more sobering knowledge that for his terminal students the senior English class may be the last chance to master language skills that will help them meet the speaking and writing demands of a lifetime."
About teaching literature vs. teaching writing: "Relatively few college students fail because of inadequate preparation in literature compared to the number who fail because they cannot write."
About expository writing: "In teaching expository writing, a teacher must deal with four kinds of composition problems; the problem of the word; the problem of the sentence; the problem of the paragraph; and the problem of the longer composition."
And "The most important and perhaps most difficult thing to achieve in teaching expository writing is good organization. Organization can be most concretely taught through the paragraph which, in small compass, demands most of the important writing skills."
Interesting to note that author John Warriner taught English in junior and senior highschools and in college. Co-author Francis Griffith had a doctoral degree in education from Columbia University, and was for many years Chairman of English and Speech in a Brooklyn, New York, High School.
Contents:
Part One: Grammar
Parts of Speech, Parts of a Sentence, Phrase, Clause
Part Two: Usage
Levels of Usage (Standard vs. Substandard English), Agreement, Pronouns, Verbs, Modifiers
Part Three: Composition: Sentence Structure
Sentence Completeness, Coordination/Subordination, Clear Reference, Placement of Modifiers, Parallel Structure (really important!), Unnecessary Shifts in Sentences, Sentence Conciseness, Sentence Variety, Effective Diction, Exercises in Sentence Revision
Part Four: Composition: Paragraphs and Longer Papers
The Effective Paragraph, Expository Writing, Language and Logic, Exercises in Composition, Research Paper, Letter Writing
Part Five: Aids to Good English
Information in the Library, Reference Books, Dictionary, Vocabulary
Part Six: Speaking and Listening
Discussion and Debate, Effective Speech
Part Seven: Mechanics
Capitalization, Punctuation, Manuscript Form, Spelling
Part Eight: A New Look at Grammar
Structural and Transformational Grammars
College Entrance and Other Examinations
Tests of Verbal Aptitude, Composition Tests
Supplement
Making Writing Interesting
From the Preface (citation above):
"The teacher of senior English occupies a difficult but challenging position. Because the course he teaches is in large part a summary of all the English courses that have preceded it, he feels obligated to review, or reteach, everything. Two thoughts impress upon him the magnitude of his responsibilities. The first is the image of the college English instructor lurking in the future of his college-bound students, ready and, it often seems, eager to find weaknesses in their high school preparation. The other is the even more sobering knowledge that for his terminal students the senior English class may be the last chance to master language skills that will help them meet the speaking and writing demands of a lifetime."
About teaching literature vs. teaching writing: "Relatively few college students fail because of inadequate preparation in literature compared to the number who fail because they cannot write."
About expository writing: "In teaching expository writing, a teacher must deal with four kinds of composition problems; the problem of the word; the problem of the sentence; the problem of the paragraph; and the problem of the longer composition."
And "The most important and perhaps most difficult thing to achieve in teaching expository writing is good organization. Organization can be most concretely taught through the paragraph which, in small compass, demands most of the important writing skills."
Interesting to note that author John Warriner taught English in junior and senior highschools and in college. Co-author Francis Griffith had a doctoral degree in education from Columbia University, and was for many years Chairman of English and Speech in a Brooklyn, New York, High School.
Monday, July 16, 2007
Units are Factors
I started going over more word problems with my son. We jumped into the DRT governing equation: Distance = Rate * Time. I wanted to show him that you can treat units like factors that are multiplied and divided.
For example, if you have 30 mph, this is really 30 * miles/hour. The 30 and the miles are in the numerator and the hour is in the denominator. You should treat them just like any other factor in the equation. You can move them around using:
a*b = b*a
and cancel them with
a/a = 1
For simple equations, like D=RT, it's probably easiest to just make sure that your units are consistent and then you can ignore units. In other words, you don't want to multiply 30 mph times 60 minutes. However, if you carefully track your units, then it will jump out at you.
D = 30 mph * 60 minutes
This is
D = 30 * miles/hour * 60 * minutes
Everything is multiplied together, so I can separately combine the numbers and units to get
D = 1800 * miles * minutes / hour
This is a correct answer.
As an interesting side note, mathematicians use a dash instead of a multiply sign when two units are multiplied side by side. They also don't show the multiply sign between the number and the units. A proper form for the answer would be
D = 1800 miles-minutes/hour
or
D = 1800 minutes-miles/hour
It may be correct technically, but the nice thing about keeping the units in the equation is that you won't forget the conversion factors.
In this case, 60 minutes = 1 hour, or 1 hour/60 minutes = 1.
[a/b = 1 if a = b]
I can multiply D by 1 without change, so
D = 1800 * miles * minutes / hour * 1 * hour/(60 * minutes)
since a*b = b*a, I can move any of the factors around in the numerator and denominator (and ignore the 1).
D = 1800/60 * miles * (minutes*hour)/(minutes*hour)
(minutes*hour)/(minutes*hour) = 1, so
D = 30 miles
My son doesn't like it when I do this. He can do the problem in his head. I tell him that I want to teach him about units while the problems are easy and not wait until he has to deal with many other things.
I will do one more problem.
100 miles = R * 2 hours
or
100 * miles = R * 2 * hours
To solve, divide both sides by 2 and divide both sides by hours.
R = 100/2 * miles/hour
R = 50 miles/hour
This (anal?) approach seems weird for simple problems, but it can really help you see if you are doing a problem correctly.
For example, if you have 30 mph, this is really 30 * miles/hour. The 30 and the miles are in the numerator and the hour is in the denominator. You should treat them just like any other factor in the equation. You can move them around using:
a*b = b*a
and cancel them with
a/a = 1
For simple equations, like D=RT, it's probably easiest to just make sure that your units are consistent and then you can ignore units. In other words, you don't want to multiply 30 mph times 60 minutes. However, if you carefully track your units, then it will jump out at you.
D = 30 mph * 60 minutes
This is
D = 30 * miles/hour * 60 * minutes
Everything is multiplied together, so I can separately combine the numbers and units to get
D = 1800 * miles * minutes / hour
This is a correct answer.
As an interesting side note, mathematicians use a dash instead of a multiply sign when two units are multiplied side by side. They also don't show the multiply sign between the number and the units. A proper form for the answer would be
D = 1800 miles-minutes/hour
or
D = 1800 minutes-miles/hour
It may be correct technically, but the nice thing about keeping the units in the equation is that you won't forget the conversion factors.
In this case, 60 minutes = 1 hour, or 1 hour/60 minutes = 1.
[a/b = 1 if a = b]
I can multiply D by 1 without change, so
D = 1800 * miles * minutes / hour * 1 * hour/(60 * minutes)
since a*b = b*a, I can move any of the factors around in the numerator and denominator (and ignore the 1).
D = 1800/60 * miles * (minutes*hour)/(minutes*hour)
(minutes*hour)/(minutes*hour) = 1, so
D = 30 miles
My son doesn't like it when I do this. He can do the problem in his head. I tell him that I want to teach him about units while the problems are easy and not wait until he has to deal with many other things.
I will do one more problem.
100 miles = R * 2 hours
or
100 * miles = R * 2 * hours
To solve, divide both sides by 2 and divide both sides by hours.
R = 100/2 * miles/hour
R = 50 miles/hour
This (anal?) approach seems weird for simple problems, but it can really help you see if you are doing a problem correctly.
Too Cool
From Tony via Darren at Right on the Left Coast
Easy Graphical Multiplication Trick - video powered by Metacafe
No, I still don't Everyday Math.
Easy Graphical Multiplication Trick - video powered by Metacafe
No, I still don't Everyday Math.
the process
from Why Johnny Can't Write:
and, today's factoid:
Apparently the idea that "writing is rewriting" has not been always with us.
No.
Prior to 1982, apparently, writing was not rewriting. Writing was writing and rewriting was rewriting. Two different things.
The idea that "writing is rewriting" comes to us from Donald Murray, who cooked it up in 1982.
NAEP 1999 Writing Report Card by state
A method for teaching writing called the "process approach" is on the increase in many school districts. Supporters of the method are admirably enthusiastic. They have publicized it widely through articles in professional journals and worked diligently to stamp out the use of other methods such as sentence combining which they call "unnatural writing" or "mechanistic." [Rousseau alert]
However, there are signs that the process approach may look better in professional articles than in practice. Recent studies show it is not particularly effective in typical school settings....
The growing use of the process approach is reflected by this statement in The Writing Report Card, the report from the National Assessment of Educational Progress on our students' writing skills:
The emphasis in writing instruction moved from the final product to the process--planning, drafting, revising, and editing. As a result, school districts across the country have begun to institute process-oriented approaches to writing instruction.
But The Writing Report Card is not able to give the process approach a high grade:
Some students did report extensive exposure to process-oriented writing activities, yet the achievement of these students was not consistently higher or lower than the achievement of those who did not receive such instruction. At all three grade levels assessed, students who said their teacheres regularly encouraged process-related activities wrote about as well as students who said their teachers did not.
and, today's factoid:
Apparently the idea that "writing is rewriting" has not been always with us.
No.
Prior to 1982, apparently, writing was not rewriting. Writing was writing and rewriting was rewriting. Two different things.
The idea that "writing is rewriting" comes to us from Donald Murray, who cooked it up in 1982.
NAEP 1999 Writing Report Card by state
first crack at editing exercise
re: eureka
wow
C. didn't have a clue how to proceed.
He only managed to cut one word:
I thought "even" needed to stay put, but I see now that the transition is implied.
This is going to be hard for me, too.
Of course I'm wondering whether I'm starting at the top. Is there a simpler way into this kind of exercise?
I will mull.
Meanwhile, on the math front, C. did the Saxon Fast Facts Multiplication sheet - 64 1-digit multiplication problems - in 2 minutes 37 seconds, getting 100% correct. That seemed good (Saxon says the sheet should be done in less than 5 minutes) until I re-read this post:
More confusion. Each "simple" multiplication problem has a 2-digit answer, whereas most simple addition problems have 1-digit answers. I think.
Tomorrow I'm going to have him do 100 simple addition problems and see what's what.
If we have to practice writing digits, we will practice writing digits.
His handwriting is terrible. I came across some of his work from a couple of years ago and discovered there's been no improvement at all.
My efforts to afterschool handwriting, which I abandoned lo these many years ago, were an abject failure.
expert advice on teaching writing from Joanne Jacobs
eureka
more from Joanne Jacobs
doctor pion on writing a precis and critical reading
home writing program in place, for now
wow
C. didn't have a clue how to proceed.
He only managed to cut one word:
Stunt people were around long before films.Even Shakespeare probably used them in fight scenes.
I thought "even" needed to stay put, but I see now that the transition is implied.
This is going to be hard for me, too.
Of course I'm wondering whether I'm starting at the top. Is there a simpler way into this kind of exercise?
I will mull.
Meanwhile, on the math front, C. did the Saxon Fast Facts Multiplication sheet - 64 1-digit multiplication problems - in 2 minutes 37 seconds, getting 100% correct. That seemed good (Saxon says the sheet should be done in less than 5 minutes) until I re-read this post:
[I]n regular classrooms we learned that students need to be able to write answers to between 70 and 90 simple addition problems per minute in order to be able to successfully and smoothly master arithmetic story problems.
More confusion. Each "simple" multiplication problem has a 2-digit answer, whereas most simple addition problems have 1-digit answers. I think.
Tomorrow I'm going to have him do 100 simple addition problems and see what's what.
If we have to practice writing digits, we will practice writing digits.
His handwriting is terrible. I came across some of his work from a couple of years ago and discovered there's been no improvement at all.
My efforts to afterschool handwriting, which I abandoned lo these many years ago, were an abject failure.
expert advice on teaching writing from Joanne Jacobs
eureka
more from Joanne Jacobs
doctor pion on writing a precis and critical reading
home writing program in place, for now
WSJ Five Best books on memory
The Wall Street Journal runs a weekly "Five Best Books on" feature, each one written by a different person.
Eric Kandel wrote the Five Best list of books on memory: ($?)
1. Ficciones
By Jorge Luis Borges
Grove, 1962
....one of the most fascinating descriptions of memory in fiction can be found in Jorge Luis Borges's seminal short-story collection, "Ficciones," first published in 1945 in Spanish. Borges, who knew for much of his life that he was slowly going blind from a hereditary disease, had a deep sense of the central and sometimes paradoxical role of memory in human existence. This sense informs much of "Ficciones" but particularly the story "Funes, the Memorious," which concerns a man who suffers a modest head injury after falling off a horse and, as a result, cannot forget anything he has ever experienced, waking or dreaming. But his brain is filled only with detail, crowding out universal principles. He can't create because his head is filled with garbage! We know that an excessively weak memory is a handicap, but, as Borges shows, having too good a memory can be a handicap as well -- the capacity to forget is a blessing.
2. Memories Are Made of This
By Rusiko Bourtchouladze
Columbia, 2002
There are several good introductions to the biology of memory storage for the general reader, but I particularly like Rusiko Bourtchouladze's. A gifted writer who is also a behaviorist, she discusses both of the great themes of memory research.....Bourtchouladze describes the now famous patient called H.M., who underwent brain surgery that left him with a devastating memory loss. H.M. could not store any new information about people, places and objects. The great Canadian psychologist Brenda Milner studied H.M. and, in a classic analysis carried out over two decades, succeeded in localizing this component of memory storage to the medial temporal lobe. Bourtchouladze brings these riveting discoveries to life.
3. Memory and Brain
By Larry R. Squire
Oxford, 1987
"Memory and Brain" is a classic in the biology of memory. In it, Larry R. Squire, a professor of psychiatry and neuroscience at the University of California at San Diego, provides a superb historical overview of the key experiments and insights that have given rise to our current understanding of the problem of memory storage. Squire himself has played a vital role in this history: He pioneered our understanding that memory exists in two major forms: declarative memory (this is the kind of memory that H.M. lost) and procedural memory (for motor and perceptual skills such as riding a bike or hitting a backhand -- this is the memory that H.M. retained).....
4. The Seven Sins Of Memory
By Daniel L. Schacter
Houghton Mifflin, 2001
In "The Seven Sins of Memory: How the Mind Forgets and Remembers," Harvard professor Daniel L. Schacter shows that declarative memory (the kind involving people, places and objects) is highly fallible and susceptible to distortion and suggestion. The seven "sins" refers to memory's various weaknesses: its transience, absentmindedness, blocking, misattribution, suggestibility, bias and persistence. Schacter .... [reveals memory's] extraordinary vulnerability to influence by authority figures....
5. Memory From A to Z
By Yadin Dudai
Oxford, 2002
Any question that remains unanswered after reading the above works by Bourtchouladze, Squire and Schacter can be answered by Yadin Dudai, a professor at the Weizmann Institute in Israel. ...The book is a handy reference, accessible to the general reader.
In Search of Memory: The Emergence of a New Science of Mind by Eric Kandel
Psychiatry, Psychoanalysis, and the New Biology of the Mind by Eric Kandel
Principles of Neural Science by Eric Kandel
Memory: From Mind to Molecules by Kandel & Squire
Eric Kandel wrote the Five Best list of books on memory: ($?)
1. Ficciones
By Jorge Luis Borges
Grove, 1962
....one of the most fascinating descriptions of memory in fiction can be found in Jorge Luis Borges's seminal short-story collection, "Ficciones," first published in 1945 in Spanish. Borges, who knew for much of his life that he was slowly going blind from a hereditary disease, had a deep sense of the central and sometimes paradoxical role of memory in human existence. This sense informs much of "Ficciones" but particularly the story "Funes, the Memorious," which concerns a man who suffers a modest head injury after falling off a horse and, as a result, cannot forget anything he has ever experienced, waking or dreaming. But his brain is filled only with detail, crowding out universal principles. He can't create because his head is filled with garbage! We know that an excessively weak memory is a handicap, but, as Borges shows, having too good a memory can be a handicap as well -- the capacity to forget is a blessing.
2. Memories Are Made of This
By Rusiko Bourtchouladze
Columbia, 2002
There are several good introductions to the biology of memory storage for the general reader, but I particularly like Rusiko Bourtchouladze's. A gifted writer who is also a behaviorist, she discusses both of the great themes of memory research.....Bourtchouladze describes the now famous patient called H.M., who underwent brain surgery that left him with a devastating memory loss. H.M. could not store any new information about people, places and objects. The great Canadian psychologist Brenda Milner studied H.M. and, in a classic analysis carried out over two decades, succeeded in localizing this component of memory storage to the medial temporal lobe. Bourtchouladze brings these riveting discoveries to life.
3. Memory and Brain
By Larry R. Squire
Oxford, 1987
"Memory and Brain" is a classic in the biology of memory. In it, Larry R. Squire, a professor of psychiatry and neuroscience at the University of California at San Diego, provides a superb historical overview of the key experiments and insights that have given rise to our current understanding of the problem of memory storage. Squire himself has played a vital role in this history: He pioneered our understanding that memory exists in two major forms: declarative memory (this is the kind of memory that H.M. lost) and procedural memory (for motor and perceptual skills such as riding a bike or hitting a backhand -- this is the memory that H.M. retained).....
4. The Seven Sins Of Memory
By Daniel L. Schacter
Houghton Mifflin, 2001
In "The Seven Sins of Memory: How the Mind Forgets and Remembers," Harvard professor Daniel L. Schacter shows that declarative memory (the kind involving people, places and objects) is highly fallible and susceptible to distortion and suggestion. The seven "sins" refers to memory's various weaknesses: its transience, absentmindedness, blocking, misattribution, suggestibility, bias and persistence. Schacter .... [reveals memory's] extraordinary vulnerability to influence by authority figures....
5. Memory From A to Z
By Yadin Dudai
Oxford, 2002
Any question that remains unanswered after reading the above works by Bourtchouladze, Squire and Schacter can be answered by Yadin Dudai, a professor at the Weizmann Institute in Israel. ...The book is a handy reference, accessible to the general reader.
In Search of Memory: The Emergence of a New Science of Mind by Eric Kandel
Psychiatry, Psychoanalysis, and the New Biology of the Mind by Eric Kandel
Principles of Neural Science by Eric Kandel
Memory: From Mind to Molecules by Kandel & Squire
Susan S on The Paragraph Book & writing a how-to
I love this -
I've been digging into the Writing Skills book from EPS, also. I have book 2.
It works well with The Paragraph Book. Book 2 is a bit more sophisticated than The Paragraph Book, so it feels more like a book pointed towards remedial upper-middle school, or high school.
It delves into formula, but is not so rigid about following a specific one like The Paragraph book.
The Writing Skills teacher's guide is not so much a book with the answers, but a good overall how-to for teachers and parents. I still like answers (even if there's more than one) when I get a teacher's guide, but this guide is really informative.
Writing Skills is very specific with its target concepts, (like The Paragraph Book,) but covers more ground in a more advanced way.
The Paragraph book looks deceptively simple, but it has revealed some interesting things about my son. For instance, book one is all about writing a simple paragraph about how to do something. They have to write several of these kinds of paragraphs with the formula: FNTF (which means First, Next, Then, Finally...)
My son wrote one on how to put toothpaste on a toothbrush. He wrote as sparingly as he could since he thought it was a stupid exercise. I kept telling him he needed more detail, but he argued that he didn't.
Finally, I had him read to me his paragraph while I tried to follow his directions exactly as though I was an alien. After bursting out laughing at my attempts to follow his directions, he finally got what I was saying.
He seems to have no sense of audience.
math facts at The Key School
Grade Four Timed Tests
Fluency with Basic Math Facts
September 11, 2006
The Key School is supposed to be one of the best private schools in the country. Based in what my friend who has two kids there tells me, it is.
Fluency with Basic Math Facts
September 11, 2006
The goal of timed tests is computational fluency: by this we mean quick and accurate knowledge of math facts. As has been said before to parents in earlier grade levels (and is worthy of repeating), automatic recall of basic math facts is desired because it frees up students’ minds for complex problem solving. To this end, fourth grade students will prepare for weekly timed tests. Below are the details.
What: Each timed tests consists of 50 problems to be completed in four minutes or less, although many students set personal goals of two minutes. Timed tests begin with subtraction facts (0-20) and, in the winter, move to multiplication facts (0-9).
When: Timed tests are given every Wednesday for the entire year. Students keep corrected timed tests in their math binders along with a chart of their progress.
How: We review study tips with students and provide work sheets for practice. Enclosed are strategies for subtraction and multiplication to help your child polish his or her math facts at home. Students can make flash cards of difficult math facts. Additionally, the Lower School’s math library has a variety of math aids available for home practice; materials may be borrowed for two weeks at a time.
The Key School is supposed to be one of the best private schools in the country. Based in what my friend who has two kids there tells me, it is.
need for speed - Vlorbik, Steve, Doug Sundseth
I'm assuming this is from Vlorbik:
All I can say to that is: don't listen to me.
Having got my "start" as a parent in ABA,* naturally I think it would be an excellent idea to introduce celeration charting into K-12.
But I have no idea - none - what the fall-out of such a scheme might be.
Here at ktm we tend to assume parents can't get too far lobbying for change in their districts. But there's always the other possibility: you spend 10 years of your life pushing, bugging, noodging, and otherwise squeaky-wheeling your way to actual change, and it turns out to be the wrong change.
Although I stumbled across the phrase "unintended consequences" relatively late in life, the instant I read it I thought: Bingo. Probably anyone who's managed to have not just one but two autistic kids has assimilated the saying about the best laid plans of mice and men often running amok.
here's Steve H:
These two comments led me to post my question to our "math brains":
How fast are you at the fundamentals?
I'm not quite sure why I ask, apart from the obvious, which is that I need some kind of rough performance standard for C & for me.
The field of reading appears to have well-developed fluency standards.
Math seems to be different. I've come across performance standards for the basic math facts, but I'm thinking about fluency on some of the "composite skills," too. I imagine the world of precision teaching has not yet produced research-based standards for, say, how quickly an expert can factor a trinomial.
Or: should there even be such a thing?
I don't know which higher-level component skills math experts typically learn to automaticity.
Here's Doug's answer to "how fast are you?":
As to the loading dock issue, I do have a serious comment: I've led speed drills with kids, and they're fun. Or at least the kids and I found them fun in our afterschool setting. A speed drill takes 5 minutes max (in my own experience 1 minute would have been better, though Saxon's sheets are 5 minutes long); the kids get a charge out of them; and everyone can see himself beating his last time. I was amazed at how quickly the kids picked up speed from one drill to the next, including one high-end SPED kid. That boy zoomed.
A timed drill wakes kids up and, as John Saxon wrote, "sets an up-tempo atmosphere to start the lesson." Or at least it did for me.
I have no idea what would happen if you introduced celeration charts in all subjects, or with all fundamentals..... but I can predict with some confidence that you aren't going to lose future math teachers over a requirement that they do one-minute speed drills at the start of a class.
I think.
update: Here's a Minnesota school that has posted fluency data for its students.
Do Your Students Really Know Their Math Facts? (pdf file)
performance standards (pdf file)
* I've linked to the new book by the Koegels who, while trained by Ivar Lovaas, are a different kettle of fish. The Koegels are our autism gurus.
please don't tell me that
you're taking "celeration" seriously.
to my eyes, it makes the already
formidable disincentives to teach
look almost pleasant.
if these guys actually
get any influence, i'll be
back on the loading dock ...
All I can say to that is: don't listen to me.
Having got my "start" as a parent in ABA,* naturally I think it would be an excellent idea to introduce celeration charting into K-12.
But I have no idea - none - what the fall-out of such a scheme might be.
Here at ktm we tend to assume parents can't get too far lobbying for change in their districts. But there's always the other possibility: you spend 10 years of your life pushing, bugging, noodging, and otherwise squeaky-wheeling your way to actual change, and it turns out to be the wrong change.
Although I stumbled across the phrase "unintended consequences" relatively late in life, the instant I read it I thought: Bingo. Probably anyone who's managed to have not just one but two autistic kids has assimilated the saying about the best laid plans of mice and men often running amok.
here's Steve H:
"Since 1990 the Standard Celeration Society has comprised a collegial organization for all persons who use Standard Celeration Charts to monitor and change human behavior frequencies."
I'm all in favor of mastery and speed in the basics of math, but I'm not too keen on schools trying to monitor units of behavior frequency change. I would rather have them monitor units of correctness (grading) on weekly quizzes and tests.
These two comments led me to post my question to our "math brains":
How fast are you at the fundamentals?
I'm not quite sure why I ask, apart from the obvious, which is that I need some kind of rough performance standard for C & for me.
The field of reading appears to have well-developed fluency standards.
Math seems to be different. I've come across performance standards for the basic math facts, but I'm thinking about fluency on some of the "composite skills," too. I imagine the world of precision teaching has not yet produced research-based standards for, say, how quickly an expert can factor a trinomial.
Or: should there even be such a thing?
I don't know which higher-level component skills math experts typically learn to automaticity.
Here's Doug's answer to "how fast are you?":
Quite fast; I normally see the answer to simple questions without consideration.
Possibly related: In the summer between 4th and 5th grade, the summer school I attended did mad-minute multiplication. We were doing 100 single-digit problems in one minute with 95%+ accuracy.
More generally, I've learned other things by extended rote practice and I still know most of those things with no real thought. (German irregular verbs, typing, judo throws.)
Yes, the practice is tedious for both student and teacher. But the student only has to do it once and the teacher is being paid very well. (Note: the work of a teacher is easier and the pay is better than the work and pay on a loading dock -- I've worked on a loading dock.)
As to the loading dock issue, I do have a serious comment: I've led speed drills with kids, and they're fun. Or at least the kids and I found them fun in our afterschool setting. A speed drill takes 5 minutes max (in my own experience 1 minute would have been better, though Saxon's sheets are 5 minutes long); the kids get a charge out of them; and everyone can see himself beating his last time. I was amazed at how quickly the kids picked up speed from one drill to the next, including one high-end SPED kid. That boy zoomed.
A timed drill wakes kids up and, as John Saxon wrote, "sets an up-tempo atmosphere to start the lesson." Or at least it did for me.
I have no idea what would happen if you introduced celeration charts in all subjects, or with all fundamentals..... but I can predict with some confidence that you aren't going to lose future math teachers over a requirement that they do one-minute speed drills at the start of a class.
I think.
update: Here's a Minnesota school that has posted fluency data for its students.
Do Your Students Really Know Their Math Facts? (pdf file)
performance standards (pdf file)
* I've linked to the new book by the Koegels who, while trained by Ivar Lovaas, are a different kettle of fish. The Koegels are our autism gurus.
Subscribe to:
Posts (Atom)